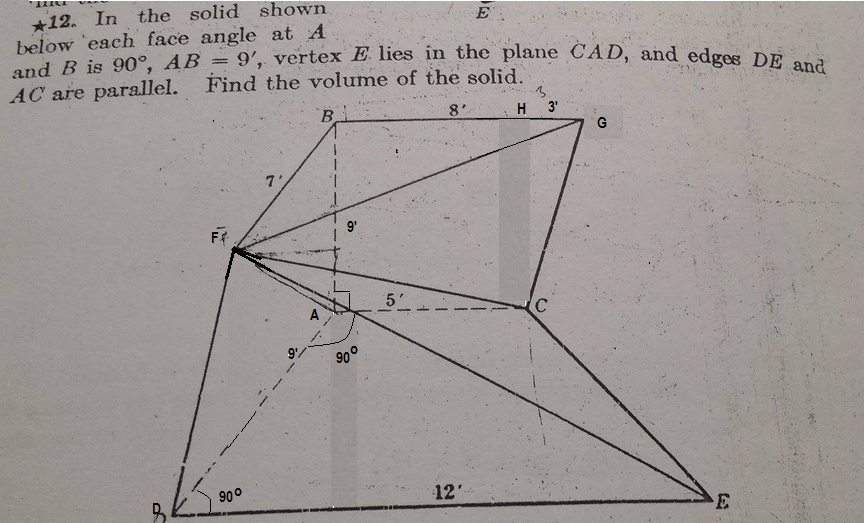
On observation of the given figure it appears that the solid is a combination of two pyramids, So the problem can be solved by using the following formula
#"Volume of a pyramid"=1/3xx"Area of its base"xx "its height"#
The base of each pyramid is a trapezium and its area is determined by the following formula
#"Area of Trapezium"#
#=1/2xx"sum of its two parallel sides"xx "distance between them"#
Pyramid - I
Its base is a Trapezium ABGC having parallel sides #BG=8'# and #AC=5'# and the distance between these sides is #AB=9'#
Again the height of this Pyramid #BF=7'# (Since each face angle at B is #90^@#)
So volume of this pyramid-I
#V_1=1/3xx"Area of its Trapezoidal base ABGC"xx "Its height "BF#
#" "=1/3xx1/2(BG+AC)xxABxxBF#
#" "=1/3xx1/2(8+5)xx9xx7ft^3=136.5ft^3#
Pyramid - II
Its base is a Trapezium ADEC having parallel sides #AC=5'# and #DE=12'# and the distance between these sides is #AD=9'#
Again the height of this Pyramid #EK=BA=9'# (Since each face angle at A is #90^@#)
So volume of this pyramid-II
#V_2=1/3xx"Area of its Trapezoidal base ADEC"xx "Its height "BA#
#" "=1/3xx1/2(DE+AC)xxADxxBA#
#" "=1/3xx1/2(12+5)xx9xx9ft^3=229.5ft^3#
So total volume of the solid
#V=V_1+V_2=(136.5+229.5)ft^3=366ft^3#


