What is the MO diagram of #"O"_2# in its ground state, and how do I calculate the bond order?
1 Answer
The ground state of oxygen molecule is the triplet state, which has two unpaired valence electrons in its

From this, you should be able to write the electronic configuration by inspection:
#overbrace(color(blue)((sigma_(1s))^2 (sigma_(1s)^"*")^2))^"deep core orbitals " overbrace(color(blue)((sigma_(2s))^2 (sigma_(2s)^"*")^2))^"outer core orbitals" underbrace(color(blue)((sigma_(2p_z))^2 (pi_(2p_x))^2(pi_(2p_y))^2 (pi_(2p_x)^"*")^1 (pi_(2p_y)^"*")^1))_"valence orbitals"#
It's exactly how you would approach the atomic configuration, with changed notation. Instead of notating atomic orbitals, we notate molecular orbitals.
As you (should) have been taught,
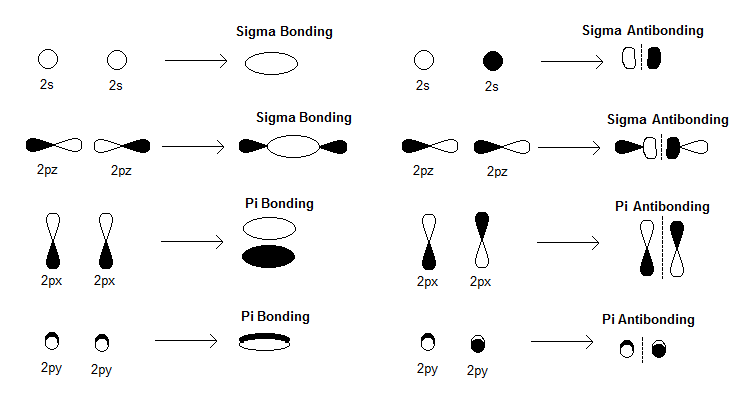
By conservation of orbitals, there must be the same number of molecular orbitals as atomic orbitals.
Its bond order is intuitive. It has a double bond, so its bond order is anticipated to be
#color(blue)("Bond Order") = 1/2 ("Bonding e"^(-) - "Antibonding e"^(-))#
#= 1/2 [underbrace(overbrace(2)^(sigma_(1s))+overbrace(2)^(sigma_(2s))+overbrace(2)^(sigma_(2p_z))+overbrace((2 xx 2))^(pi_(2p_(x//y))))_("Bonding e"^(-)'s) - (underbrace(overbrace(2)^(sigma_(1s)^"*")+overbrace(2)^(sigma_(2s)^"*")+overbrace((2 xx 1))^(pi_(2p_(x//y)^"*")))_("Antibonding e"^(-)'s))]#
#= color(blue)(2)#

