First determine the molar mass of fluorine gas #("F"_2")#, then determine the moles of fluorine gas. The molar mass of fluorine is its atomic mass from the periodic table in g/mol.
Molar Mass of #"F"_2"#
Multiply the molar mass of #"F"# times the subscript 2 in the formula for fluorine gas, #"F"_2"#.
#M("F"_2")##=##(2 xx "18.998 g/mol")="37.996 g/mol"#
Moles #"F"_2"#
Divide the given mass by the molar mass.
#"mol F"_2"##=##(15.0cancel"g")/(37.996cancel"g"/"mol")="0.39478 mol F"_2"#
I am keeping a couple of guard digits to reduce rounding errors. The final answer will be rounded to three significant figures.
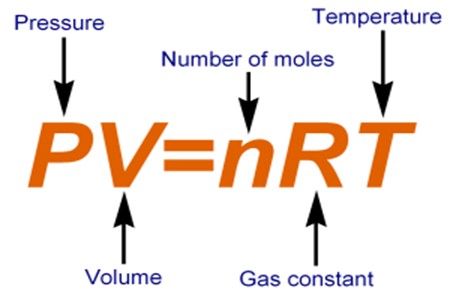
Use the formula for the ideal gas law to determine the volume of the fluorine gas.
#"Current STP"##=##"0"^@"C"# or #"273.15 K"# (for gases) and #"100 kPa"#
#R="8.3144598 L kPa K"^(-1) "mol"^(-1)#
https://en.wikipedia.org/w/index.php?title=Gas_constant&oldid=729635962
Rearrange the formula to isolate volume, substitute the known values into the formula and solve for volume.
#V=(nRT)/P#
#V=((0.39478cancel"mol")xx(8.3144598 "L" cancel"kPa" cancel "K"^(-1) cancel"mol"^(-1))xx(273.15cancel"K"))/(100"kPa")="8.97 L"# (rounded to three significant figures)
If your teacher still uses #"STP"##=##"0"^@"C"# or #"273.15 K"# and #"1 atm"#, switch #"0.082057338 L atm K"^(-1) "mol"^(-1)"# for the gas constant #R#, and #"1 atm"# for pressure #P# in the equation. The volume will be #"8.58 L"#.
