Question #5baa9
1 Answer
Feb 10, 2017
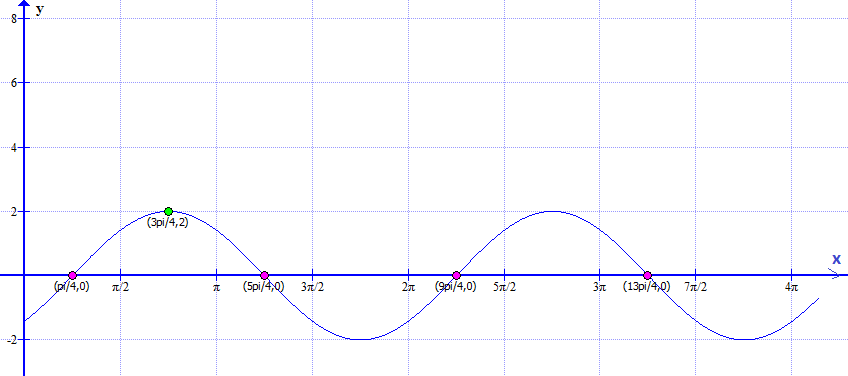
Given
To get x-intercepts we can put y=0 and solve for
So
Putting
Putting
Putting
Putting
(a) So points of x-intercepts over
b) For maximum value of y the value of
So
For
The point(in (0,2pi) where the graph of this function reaches
maximum is