Question #3b185

Question

1192 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2016-11-17T13:05:27

See below.

Calling #f(x) = x-elog_ex# it's minimum is located at

#(df)/(dx)=1-e/x=0#

or at #x = e#

At this point we have

#f(e)=0#

To qualify this stationary point we compute

#(d^2f)/(dx^2) =e/x^2# and for #x=e# we have

#(d^2f)/(dx^2)=e^(-1) > 0# so #x=e# represents a minimum of #f(x)#

The conclusion is:

#e log_ex le x # for #x > 0# and also #e log_ex# and #x# osculate at #x = e#.

Note that for #x < 0#, #log_ex# is not defined as a real function of a real variable.

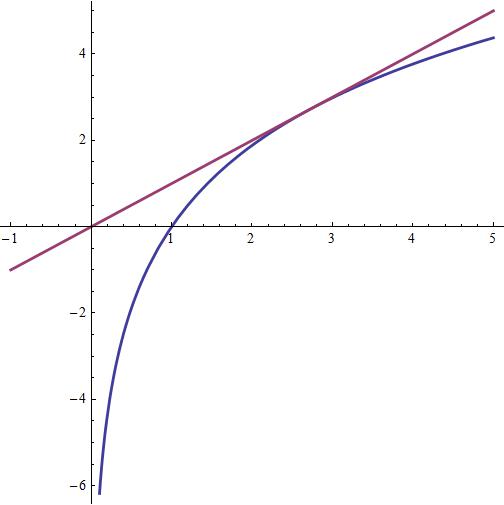
Attached a plot with #elog_ex# (blue) and #x# (red)

enter image source here