Question

Question #e6569

Answer 1

If the point `C` lies on the line `y=2x` then it will have the general coordinate `(x,2x)`.

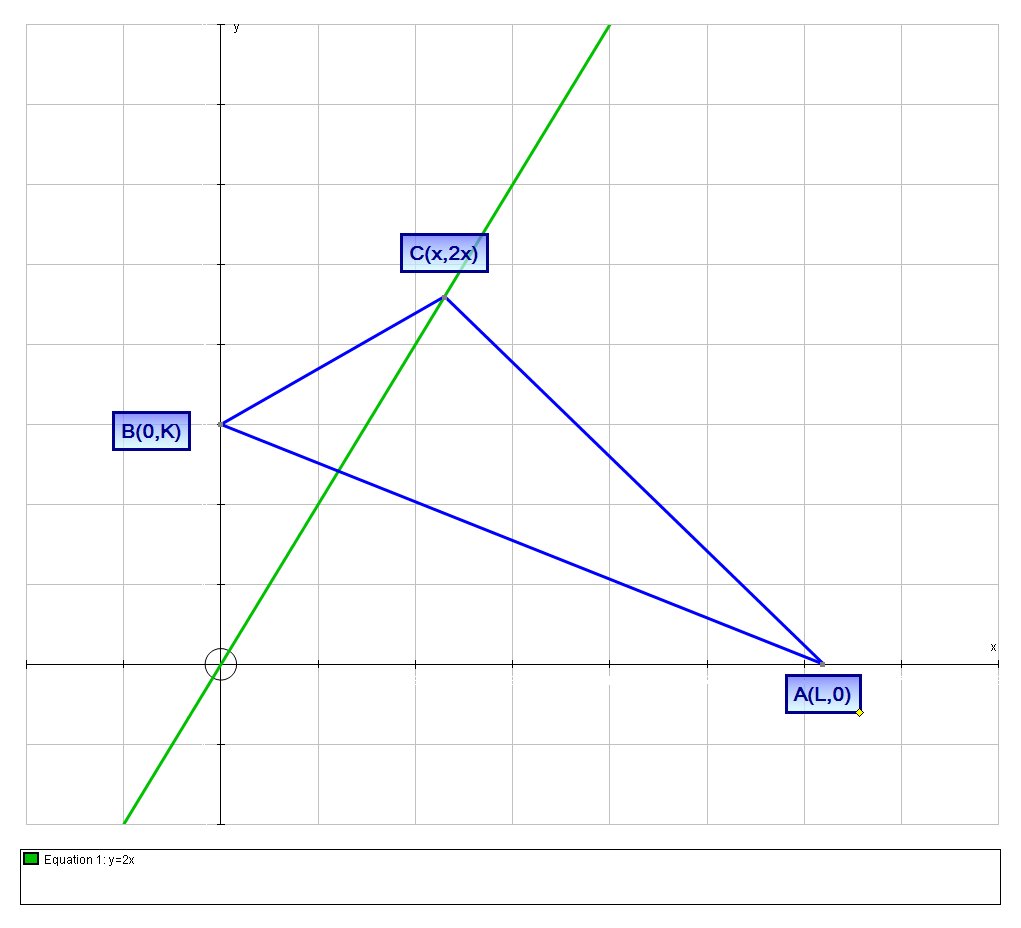
We have all three coordinates of the triangle `ABC`, namely:

`A(L,0)`, `B(0,K)` and `C(x,2x)`

The area of the triangle can then be calculated using the
shoelace formula:

`A=1/2|(x_A−x_C)(y_B−y_A)−(x_A−x_B)(y_C−y_A)|`

So we get:

`\ \ \ \ \ a=1/2|(L-x)(K-0)-(L-0)(2x-K)|`
`:. a=1/2|(L-x)K-L(2x-K)|`
`:. a=1/2|LK-Kx-2Lx+LK|`
`:. a=1/2|-Kx-2Lx|`
`:. a=1/2|-1(Kx+2Lx)|`
`:. a=1/2|Kx+2Lx|`

We are told that both `K` and `L` are positive so providing `x` is also positive (wlog) then:

`\ \ \ \ \ a=1/2(Kx+2Lx)`
`:. a=1/2Kx+Lx`

And differentiating wrt `x` gives the required result:

`\ \ (da)/dx=1/2K+L " "` QED