Question #5a331
1 Answer
The acceleration of the refrigerator is
Explanation:
We are given that
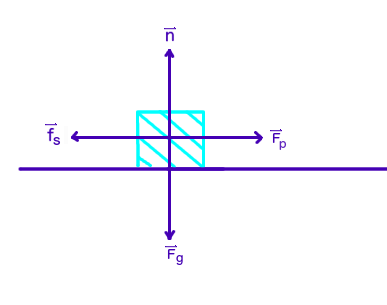
Where
#vecn# is the normal force,#vecF_g# is the force of gravity,#vecf_s# is the force of static friction, and#vecF_p# is the force of the push applied to the refrigerator. I have defined to the right as the positive direction, i.e. all force vectors pointing to the right are positive and those pointing to the left are negative. As usual, force vectors pointing up are positive, and those pointing down are negative.(Note that not all of the vector magnitudes are necessarily drawn to scale)
We can construct statements for the net force in both the parallel
#sumvecF_x=vecF_p-vecf_s#
#sumvecF_y=vecn-vecF_g#
Because the refrigerator does not move in the perpendicular direction (up or down), the net force
We know that the equation for the maximum static friction is given by
In order for the refrigerator to move, or to accelerate, we must overcome the force of static friction. Therefore, we will calculate the maximum force of static friction.
From our statement of the net force, we see that
#=(0.25)(100.0kg)(9.8m/s^2)#
#=245N# .
We must apply a force
Now with a value for the force, we can calculate the acceleration of the refrigerator. Once we overcome static friction, we have:
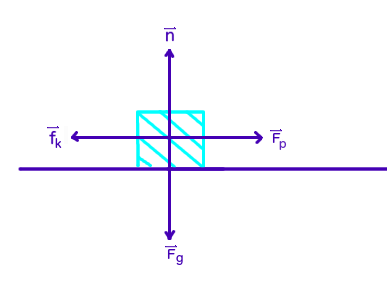
This is very similar to what we had above, except now we have the force of kinetic friction, as the object has begun to move.
Now we want to calculate
Solve for
Using our given values and what we calculated for

