Question

Question #3d4ae

Answer 1

`(216pi)/5`

Explanation:

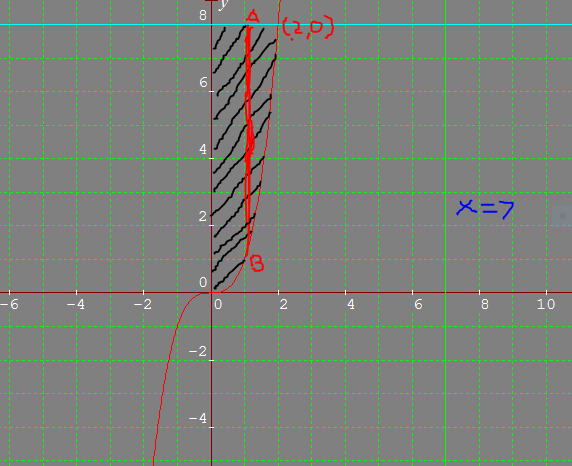
The region to be revolved around x=7 is shown shaded in black in the attached diagram. For this region x would vary from x=0 to x=2. Now consider an element of length AB(thickness dx ), of this region at a distance x from from y-axis. Length AB would be `8-x^3` and its distance from x=7 would be 7-x.

Now if this element is rotated about x=7, the surface area of this cylindrical shell would be `2pi (7-x)x^3` and its volume would be `2pi(7-x)(8-x^3) dx`

The volume of the solid so generated by revolving the whole shaded region would then be

`int_0^ 2 2pi (7-x)(8-x^3) dx`

=`2piint_0^2 (56-8x-7x^3+ x^4) dx`

=`2pi [56x-8x^2/2-7x^4 /4 +x^5/5]_0^2`

=`2pi(112-16-28 + 32/5)= 2pi(68+32/5)`

`=2pi(372/5)=(744pi)/5`