Question

Given that `cotx = 2/5`, then what is the value of `tan(2x)`, `cos(2x)` and `sin(2x)`?

Answer 1

`tan2x =-20/21`
`sin 2x \ = 20/29`
`cos 2x \ = -21/29`

Explanation:

`cotx=2/5 => tanx=5/2`

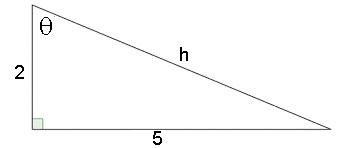
Consider the following right angle triangle:

By elementary trigonometry we have:

`tan theta = "opp"/"adj" = 5/2 => theta = x`

By Pythagoras:

`\ \ \ \ h^2 = 2^2+5^2`
`:. h^2 = 4+25`
`:. h^2 = 29`
`:. \ \h = sqrt(29)`

And so;

`sin x = "opp"/"hyp" = 5/h = 5/sqrt(29)`
`cos x = "adj"/"hyp" = 2/h = 2/sqrt(29)`

Using the identity `tan(2theta) -= (2tan theta)/(1-tan^2theta)` we have:

`tan2x = (2tan x)/(1-tan^2 x)`
`" " = (2*5/2)/(1-(5/2)^2)`
`" " = 5/(1-25/4)`
`" " = 5/(-21/4)`
`" " = -20/21`

And similarly using `sin2x=2sinxcosx`

`sin 2x = 2*5/sqrt(29)*2/sqrt(29)`
`" " = 20/29`

And similarly using `cos2x=cos^2x-sin^2x`

`cos 2x = (2/sqrt(29))^2 - (5/sqrt(29))^2`
`" " = 4/29 - 25/29`
`" " = -21/29`