#220# cars are rented at #$30# per day and for each dollar increase, #5# fewer cars are rented. What is the maximum possible income and the rent at which this maximizes?
2 Answers
Maximum income of
Explanation:
Let number of cars rented at a rate of
As
at
or
and this will maximized when
as
Hence, revenue is maximized when rate is
and revenue is
TR is maximum when 185 cars are rented out.
Revenue maximising daily rent = 37
Maximum Revenue
Explanation:
We can form a demand curve using the given information.
Daily Rent is measured along the Y - axis. We shall have Daily Rate as Price and symbolize it as
Number of cars is measured along the X - axis. Let us symbolize it as
We shall develop the AR function [Demand function]
#q_1=220#
#p_1=30#
#q_2=215#
#p_2=31#
#(p-p_1)=(p_2-p_1)/(q_2-q_1)(q-q_1)#
#p-30=(31-30)/(215-220)(q-220)#
#p-30=-1/5(q-220)#
#p-30=-1/5q+44#
#p=-1/5q+44+30#
#p=-1/5q+74# [AR function]
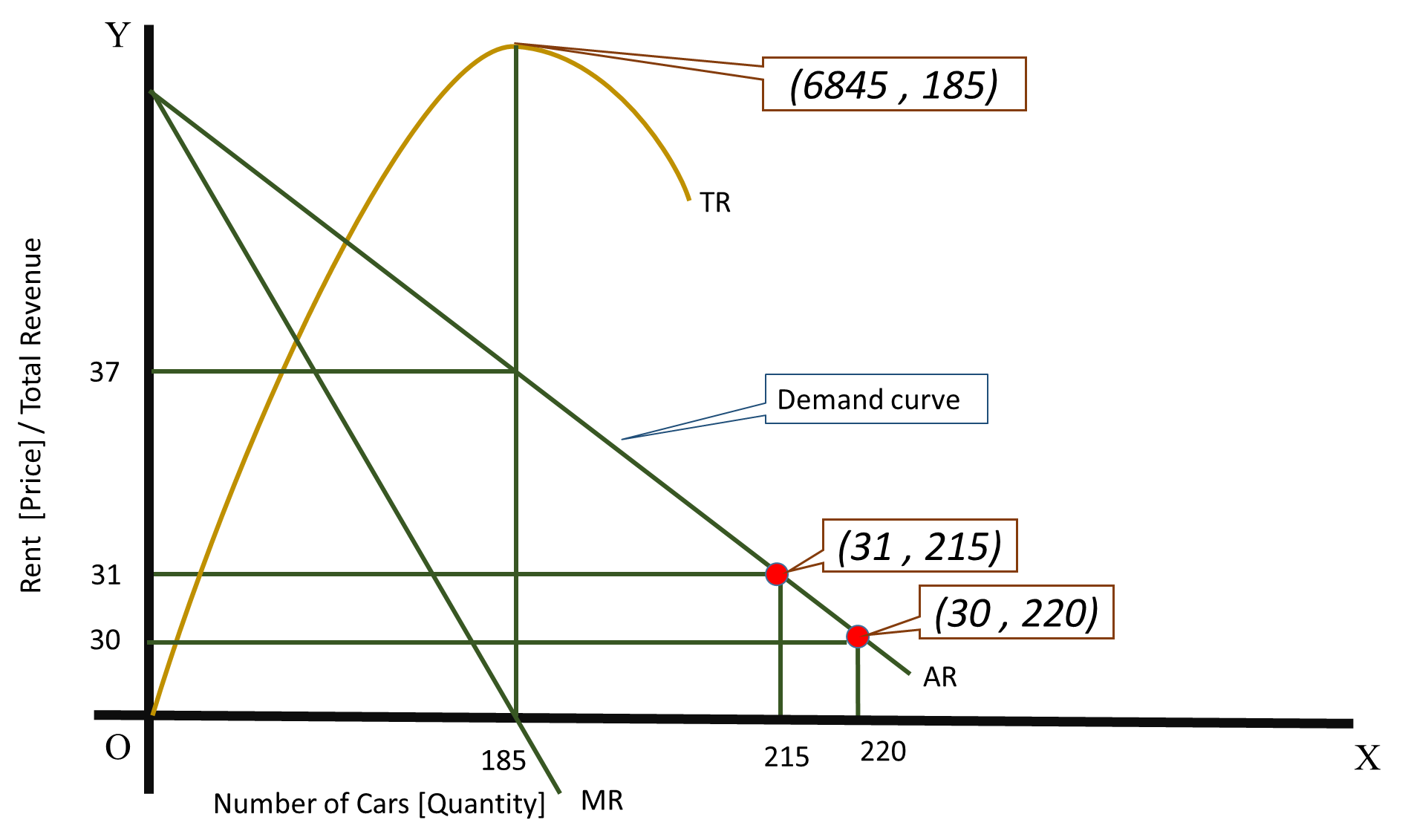
Since AR curve is downward sloping and linear, the slope of the MR curve is double the slope of AR curve. Using this piece of information let us form the MR function.
MR
MR
Total Revenue is Maximum when MR = 0.
#-2/5x+74=0#
#x=-74xx(-5/2)=370/2=185#
TR is maximum when 185 cars are rented out.
To find the price, substitute
AR
AR
Revenue maximising daily rent = 37
Maximum Revenue = Number of cars
Maximum Revenue =185

