Question

Question #27e1c

Answer 1

`0`

Explanation:

Your goal here is to figure out if `"9.9 eV"` of energy are enough to excite a hydrogen atom in its ground state and if so, the highest energy level that the electron can reach.

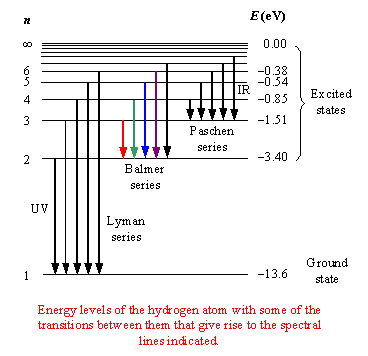
The easiest way you have of figuring out if `"9.9 eV"` is enough energy to bump the electron from the first energy level is to subtract the energy of the ground level, for which `n=1`, and the energy of the first excited state, for which `n=2`.

You will end up with--you can ignore the minus signs for this purpose

`"13.6 eV " - " 3.40 eV" = "10.2 eV"`

This tells you that in order for an electron to move from `n=1` to `n=2` in a hydrogen atom, it must absorb `"10.2 eV"` of energy.

Since you only have `"9.9 eV"` available, you can say that the electron won't absorb the energy needed for this transition to take place, which implies that the number of spectral lines emitted will be equal to `0`.

Alternatively, you can use the fact that the quantized energy levels that the electron can occupy in a hydrogen atom are described by the following equation

`E_n = -"13.6 eV"/n^2`

Here `n` represents the principal quantum number that describes a given energy level.

In your case, you will have

`E_n = -"13.6 eV" + "9.9 eV" = -"3.7 eV"`

This means that `n` will be

`n^2 = (color(red)(cancel(color(black)(-)))13.6 color(red)(cancel(color(black)("eV"))))/(color(red)(cancel(color(black)(-)))3.7color(red)(cancel(color(black)("eV")))) implies n= sqrt(13.6/3.7) ~~ 1.9`

Since you have `1 < n < 2`, you can conclude that the electron did not absorb enough energy to get to the second energy level `->` no spectral lines will be emitted.