Question #803c4
1 Answer
Theoretical Yield = Zero ... The reaction proposed does not have a driving force for formation of
Explanation:
The reaction specified is defined as a Metathesis or Double Replacement Reaction. This reaction takes the empirical form of AX + BY => AY + BX, where one of the product compounds must be a molecular structure that ‘leaves’ the reaction environment as an insoluble precipitate, soluble weak electrolyte (i.e., weak acid or weak base) or a gas decomposition product from a weak electrolyte. The substance leaving the reaction environment is generally referred to as 'The Driving Force' of the reaction. This compound prevents the reaction from reversing and reforming reactants.
To completely understand this issue, one needs to review ...
- solubility rules of ionic compounds in aqueous media,
- formation of weak acids and weak bases specifically described by the Bronsted-Lowry Theory of Acids and Bases and
- gas decomposition products formed from decomposition of labile (easy to break down) weak acids and bases formed in metathesis reactions.
A more suitable example would be the reaction of 2 grams of
Since Silver Chloride has an extremely low solubility in water, then the formation of
Example: Given ...
The balanced equation is ...
Strategy:
Convert given mass values to moles (divide by formula wt) and determine limiting reagent (if there is one) by dividing mole values by respective coefficients. The smaller number will be the limiting reagent. Apply moles of limiting reagent to equation reaction ratios to determine the moles of each product produced. Convert moles of product produced to grams by multiplying by formula weight. If a "Laboratory Yield" is available, then calculate %Yield.
Determine Limiting Reagent:
Since
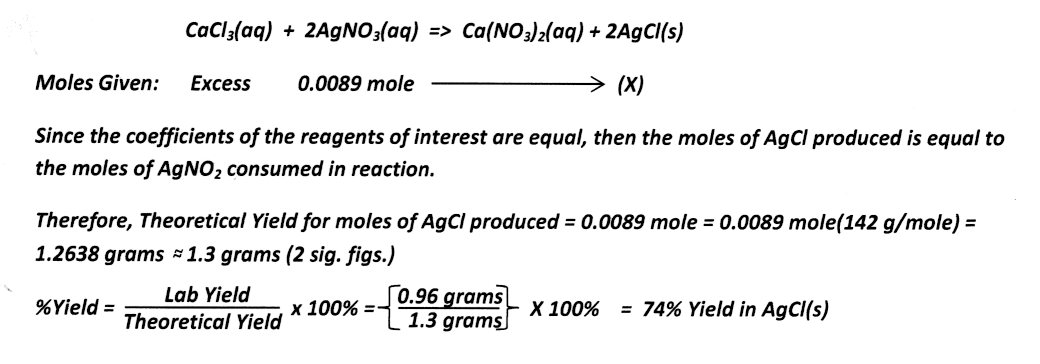
Calculating Theoretical and Percent Yield:
To complete this illustration, assume that the actual lab yield was measured to be 0.96 grams AgCl(s) recovered. Calculate the Theoretical and % Yield of AgCl.