How do you calculate the ionization energy of a hydrogen atom in its ground state?
1 Answer
Explanation:
!! VERY LONG ANSWER !!
Start by calculating the wavelength of the emission line that corresponds to an electron that undergoes a
This transition is part of the Lyman series and takes place in the ultraviolet part of the electromagnetic spectrum.
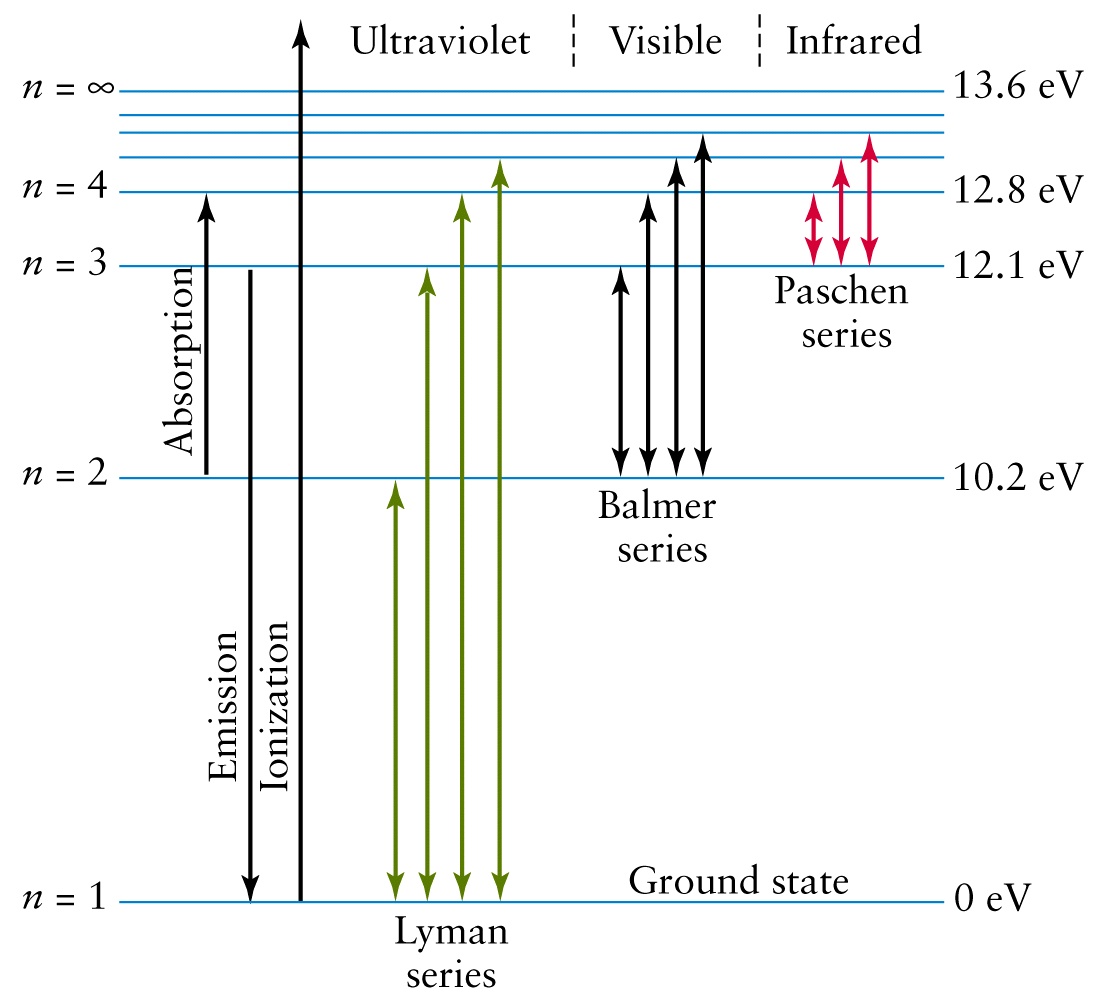
Your tool of choice here will be the Rydberg equation for the hydrogen atom, which looks like this
#1/(lamda_"e") = R * (1/n_1^2 - 1/n_2^2)#
Here
#lamda_"e"# is the wavelength of the emitted photon (in a vacuum)#R# is the Rydberg constant, equal to#1.097 * 10^(7)# #"m"^(-1)# #n_1# represents the principal quantum number of the orbital that is lower in energy#n_2# represents the principal quantum number of the orbital that is higher in energy
In your case, you have
#{(n_1 = 1), (n_2 = oo) :}#
Now, you know that as the value of
#1/n_2^2 -> 0#
This implies that the Rydberg equation will take the form
#1/(lamda) = R * (1/n_1^2 - 0)#
#1/(lamda) = R * 1/n_1^2#
which, in your case, will get you
#1/(lamda) = R * 1/1^2#
#1/(lamda) = R#
Rearrange to solve for the wavelength
#lamda = 1/R#
Plug in the value you have for
#lamda = 1/(1.097 * 10^(7)color(white)(.)"m") = 9.116 * 10^(-8)# #"m"#
Now, in order to find the energy that corresponds to this transition, calculate the frequency,
#color(blue)(ul(color(black)(nu * lamda = c)))#
Here
#nu# is the frequency of the photon#c# is the speed of light in a vacuum, usually given as#3 * 10^8# #"m s"^(-1)#
Rearrange to solve for the frequency and plug in your value to find
#nu * lamda = c implies nu = c/(lamda)#
#nu = (3 * 10^(8) color(red)(cancel(color(black)("m"))) "s"^(-1))/(9.116 * 10^(-8)color(red)(cancel(color(black)("m")))) = 3.291 * 10^(15)# #"s"^(-1)#
Finally, the energy of this photon is directly proportional to its frequency as described by the Planck - Einstein relation
#color(blue)(ul(color(black)(E = h * nu)))#
Here
#E# is the energy of the photon#h# is Planck's constant, equal to#6.626 * 10^(-34)"J s"#
Plug in your value to find
#E = 6.626 * 10^(-34)color(white)(.)"J" color(red)(cancel(color(black)("s"))) * 3.291 * 10^(15) color(red)(cancel(color(black)("s"^(-1))))#
#E = 2.181 * 10^(-18)# #"J"#
This means that in order to remove the electron from the ground state of a hydrogen atom in the gaseous state and create a hydrogen ion, you need to supply
This means that for
#"H"_ ((g)) + 2.181 * 10^(-18)color(white)(.)"J" -> "H"_ ((g))^(+) + "e"^(-)#
Now, the ionization energy of hydrogen represents the energy required to remove
To convert the energy to kilojoules per mole, use the fact that
You will end up with
#6.022 * 10^(23) color(red)(cancel(color(black)("photons")))/"1 mole photons" * (2.181 * 10^(-18)color(white)(.)color(red)(cancel(color(black)("J"))))/(1color(red)(cancel(color(black)("photon")))) * "1 kJ"/(10^3color(red)(cancel(color(black)("J"))))#
# = color(darkgreen)(ul(color(black)("1313 kJ mol"^(-1))))#
You can thus say that for
#"H"_ ((g)) + "1313 kJ" -> "H"_((g))^(+) + "e"^(-)#
The cited value for the ionization energy of hydrogen is actually

My guess would be that the difference between the two results was caused by the value I used for Avogadro's constant and by rounding.
#6.02 * 10^(23) -> "1312 kJ mol"^(-1)" vs "6.022 * 10^(23) -> "1313 kJ mol"^(-1)#

