Question

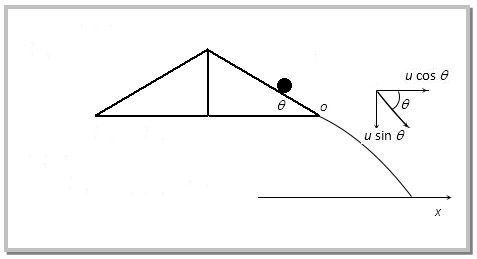
Question #96f87

Answer 1

`h = 67.6 \ m`

Explanation:

For Physics or Mechanics you should learn the "suvat" equations for motion under constant acceleration:

#{: (v=u+at, " where ", s="displacement "(m)), (s=ut+1/2at^2, , u="initial speed "(ms^-1)), (s=1/2(u+v)t, , v="final speed "(ms^-1)), (v^2=u^2+2as, , a="acceleration "(ms^-2)), (s=vt-1/2at^2, , t="time "(s)) :} #

Let us define the following variables:

# { (theta=30^o, "= Angle of projection to the horizontal", ""^o), (U, "= Speed at moment hammer leaves roof", ms^-1), (h, "= Height of the roof lip", m), (T, "= Time for hammer to hit ground after leaving roof", s) :} #

|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

INITIAL MOTION - SLIDING FROM ROOFTOP

We can apply the suvat equations parallel to the roof to find the velocity of the hammer just before it leaves the rooftop.

# { (s=,2.8,m), (u=,0,ms^-1), (v=,U,ms^-1), (a=,1.3,ms^-2), (t=,"Not Required",s) :} #

Applying `v^2=u^2+2as` we have:

`U^2 = 0 + 2(1.3)(2.8)`
`\ \ \ \ \ = 7.28`

`:. U = sqrt(7.28)`

|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

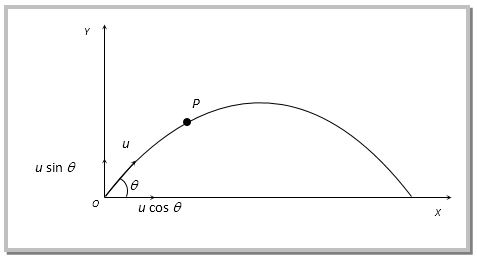
SECONDARY MOTION - FALLING UNDER GRAVITY

Horizontal Motion

The projectile will move under constant speed (NB we can still use "suvat" equation with `a=0`). We must resolve the initial speed into its horizontal component, giving us `Ucostheta`. We know it travels `3.2m` in time `T`

# { (s=,3.2,m), (u=,Ucostheta ,ms^-1), (v=,"Not Required",ms^-1), (a=,0,ms^-2), (t=,T,s) :} #

Applying `s=ut+1/2at^2` we have:

`3.2 = Ucostheta T + 0`

`:. 3.2 = sqrt(7.28)cos30^o T`

`:. 3.2 = sqrt(7.28)sqrt(3)/2 T`

`:. T = 6.4 /( sqrt(7.28)sqrt(3) )`

|||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||||

Vertical Motion

The projectile travels under constant acceleration due to gravity.

We must resolve the initial speed into its vertical component giving us `Usintheta`. We know it travels `hm` in time `T`

# { (s=,h,m), (u=,Usintheta ,ms^-1), (v=,"Not Required",ms^-1), (a=,g,ms^-2), (t=,T,s) :} #

Applying `s=ut+1/2at^2` we have:

`h = (Usintheta)(6.4 / ( sqrt(21.84) )) +1/2(g)(6.4 / ( sqrt(21.84) ))^2`
`\ \ = (sqrt(7.28))(1/2)(6.4 / ( sqrt(7.28)sqrt(3) )) +1/2(g)(6.4 / ( sqrt(7.28)sqrt(3) ))^2`
`\ \ = (3.2 / ( sqrt(7.28)sqrt(3) )) +1/2(g)( 40.96 /3 )`

`\ \ = (3.2 / ( sqrt(7.28)sqrt(3) )) +( 40.96 /6 )g`

And, if we take `g=9.8` we have:

`h = 67.586 ...`
`\ \ = 67.6 \ m`