Question

A `5 L` container holds `15` mol and `6` mol of gasses A and B, respectively. Every three of molecules of gas B bind to two molecule of gas A and the reaction changes the temperature from `360K` to `210K`. By how much does the pressure change?

Answer 1

The pressure changed by `8.03 × 10^6 Pa`.

Explanation:

To get started we’ll work out how many moles of gas are present at the end of the reaction. There are 6 moles of gas B. In the reaction gas B bonds with gas A at a ratio of 3:2 molecules, so that ratio will apply to the number of moles also. 3 moles of gas B will bond with 2 moles of gas A. In total all 6 moles of gas B will bond with 4 moles of gas A and the product of that reaction will be 2 moles of a new gas. Let’s call the new gas, gas C.

That leaves us with 15 – 4 = 11 moles of gas A, 0 moles of gas B (it all reacted), and 2 moles of gas C. So the final number of moles of gas will be 13 moles. The initial number for moles was 15 + 6 = 21 moles.

Calculate the Final Pressure
To solve the problem of the pressure change we will use the ideal gas equation:
`(pV)/(nT) =` constant.
You may be familiar with the equation stated as: `pV = nRT` where R is the molar gas constant.

Since the LHS of the equation is equal to a constant we can now state the equation like this:
`(p_1V_1)/(n_1T_1) = (p_2V_2)/(n_2T_2)`

The volume does not change so `V_1=V_2` and they will cancel out of the equation. We need to find the final pressure so rearrange for `p_2`:
`p_2 = p_1(V_1n_2T_2)/(V_2n_1T_1) = p_1(n_2T_2)/(n_1T_1)`

The above equation will give us p₂ as a multiple of p₁. Now substitute the values into the equation:
`p_2 = p_1(13 × 210)/(21 × 360) = 0.3611 p_1`

Pressure Change
To calculate by how much the pressure changes find the difference between initial and final pressures:
`p_1 – p_2 = p_1 – 0.3611 p_1 = 0.639 p_1`

Calculate the value of p₁ by using the ideal gas equation:
Volume conversion: `V = 5 L = 5 × 10^(-3) m³`

`p_1V_1 = n_1RT_1 ⇒ p_1 = (n_1RT_1) / V_1 = (21 × 8.31 × 360) / (5 × 10^(-3)) = 12.565 × 10^6 Pa`

The pressure therefore changed by:
`0.639 × 12.565 × 10^6 = 8.03 × 10^6 Pa`.

Answer 2

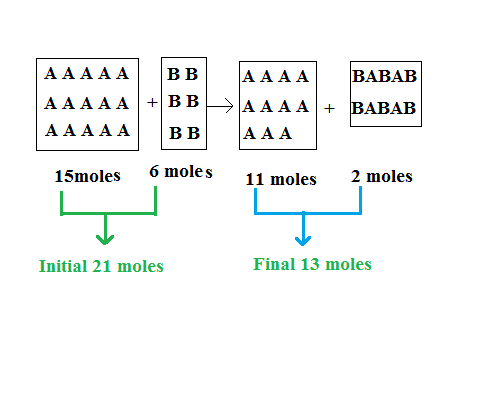
As explained in the above figure
Initial number of moles of gas molecules was `n_1=21`moles
and Final number of moles of gas molecules was `n_2=13` moles

We Know from Equation of state for ideal gas

`PV = nRT`,

where:

• P = pressure in atm
• V = volume in L
• T = temperature in K
• n = number of moles
• R = universal gas constant.=0.082`LatmK^-1mol^-1`

For Initial state
If pressure is `P_1`
Volume `V =5L`
Number of moles `n_1=21`
Temperature `T_1=360K`
and then `P_1=(n_1RT_1)/V`

For Final state
If pressure is `P_2`
Volume `V =5L`
Number of moles `n_2=13`
Temperature `T_2=210K`
and then `P_2=(n_2RT_2)/V`

So decrease in Pressure:

`= P_1-P_2=R/Vxx(n_1T_1-n_2T_2)`
`= 0.082/5xx(21xx360-13xx210)` `atm`
`= 0.082/5xx4830` `atm=79.212` `atm`
`= 79.212` `atm`

When we convert this to `Pa`, we get:

`79.212` `cancel(atm)xx101.325xx10^3` `(Pa)/cancel(atm)`

`~~ 8026xx10^3` `Pa`