Question

A balanced lever has two weights on it, the first with mass `6 kg` and the second with mass `7 kg`. If the first weight is `4 m` from the fulcrum, how far is the second weight from the fulcrum?

Answer 1

`x ~~3.429 metres`

Explanation:

`color(blue)("Comment")`
As we are determining the distance it does not matter what units of weight we use as long as they are consistent.

I am also going to demonstrate how to deal with the units of measurement. If you deal with 'Statics' to any extent then manipulating units is exceptionally important!

'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~

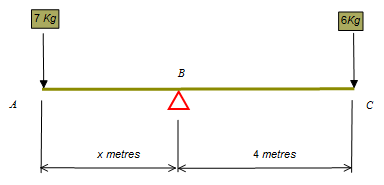
If the moments do not match then it means that the system is in motion. We can use this fact to determine the moment arms needed to establish equilibrium.

A Moment is the number resulting from an applied force multiplied by its distance to a point of rotation ( actual or theoretical).
'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)("Taking Moments about the fulcrum B")`

Let clockwise moment be positive.
Let anticlockwise moments be negative

Then for equilibrium to exist

`(+(6xx4) Kgm) +(-(7xx x) Kgm)=0`

`color(brown)(":::::::::::: Units are very important:::::::::::::::")`

`color(brown)("Note that weight"xx"distance"->Kgxxm->Kgm`

`color(brown)( "::::::::::::::::::::::::::::::::::::::::::::::::::::::::::::::::::::::")`

For this to be true then:

`24 Kgm=7x Kgm`

'~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
`color(blue)("To find the value of "x)`

To find `x` divide both sides by `7Kg`: Note I have included the units

`24/7color(white)(.) (cancel(Kg)m)/cancel(Kg) = (cancel(7Kg))/(cancel(7Kg))xx xm`

`x ~~3.429 metres` to 3 decimal places