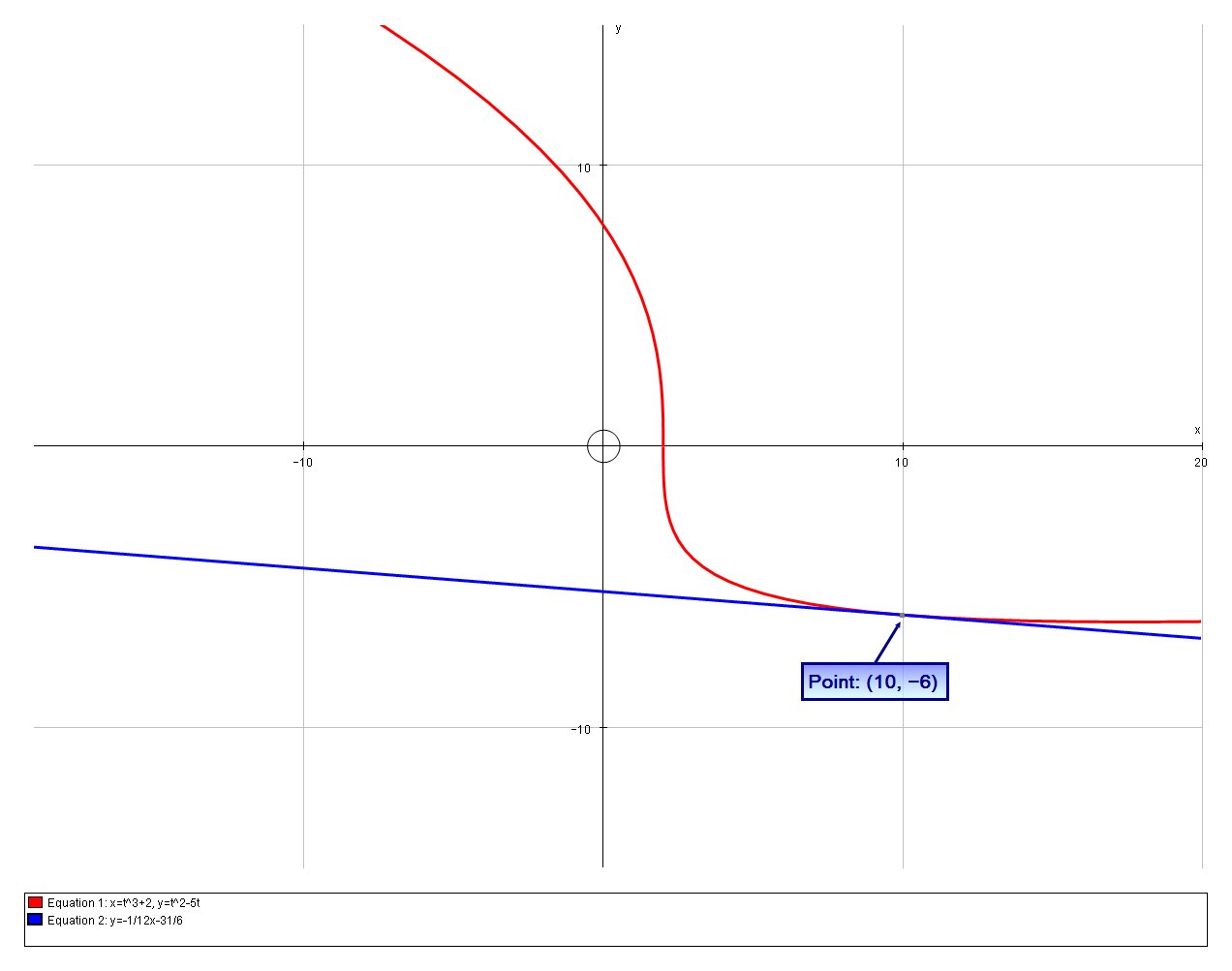
A curve in the xy-plane is defined by the parametric equations #x = t^3 + 2# and #y = t^2 - 5t# how do you find the slope of the line tangent to the curve at the point where x = 10?
1 Answer
The slope of the tangent is
Explanation:
The curve is defined by the parametric equations:
# { (x=t^3+2), (y=t^2-5t) :} #
When
# x=t^3+2 => 10=t^3+2 #
# :. t^3=8 #
# :. \ \ t=2 #
When
# y=t^2-5t => y=4-10 = -6 #
So the coordinate of interest with
We now need the derivative to establish the gradient of the tangent:
# x=t^3+2 \ => dx/dt=3t^2 #
# y=t^2-5t => dy/dt=2t-5 #
By the chain rule we have:
# dy/dx = (dy/dt)/(dx/dt) #
# " " = (2t-5)/(3t^2) #
So when
# dy/dx = -1/12 #
So our tangent has slope
# y-(-6)=-1/12(x-10) #
# :. y+6=-1/12x+10/12 #
# :. y=-1/12x-31/6 #
We can confirm this graphically: