Question

A gas occupies a volume of 0.2 L at 25 kPa. What volume will the gas occupy at 2.5 kPa?

Answer 1

`"2 L"`

Explanation:

Right from the start, assuming, of course, that the temperature and the number of moles of gas remain unchanged, you can say that the volume of the gas will increase as pressure changes from `"25 kPa"` to `"2.5 kPa"`.

That is the case because when the temperature and the number of moles of gas remain constant, the pressure of a gas varies indirectly with its volume, as described by Boyle's Law.


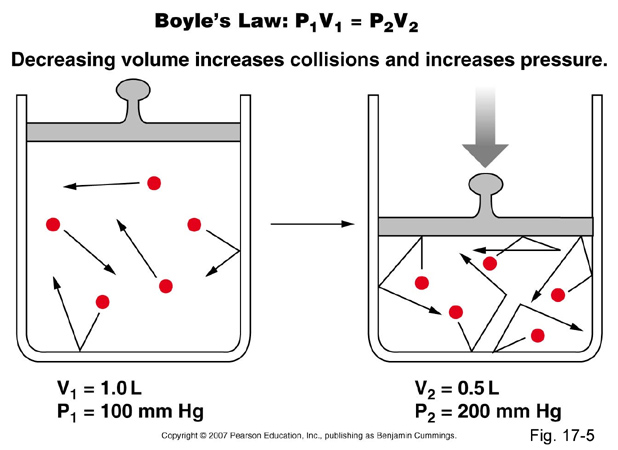
In other words, when pressure decreases by factor `k`, volume increases by the same factor `k`.

`P * V = k`

This implies that you can write

`color(blue)(ul(color(black)(P_1V_1 = P_2V_2)))`

Here

• `P_1` and `V_1` represent the pressure and volume of the gas at an initial state
• `P_2` and `V_2` represent the pressure and volume of the gas at a final state

Rearrange to solve for `V_2`

`P_1V_1 = P_2V_2 implies V_2 = P_1/P_2 * V_1`

Plug in your values to find

`V_2 = (25 color(red)(cancel(color(black)("kPa"))))/(2.5color(red)(cancel(color(black)("kPa")))) * "0.2 L" = color(darkgreen)(ul(color(black)("2 L")))`

The answer is rounded to one significant figure, the number of significant figures you have for the initial volume of the gas.

As predicted, the volume of the gas increased as a result of the decrease in pressure. Moreover, it increased by the same factor!