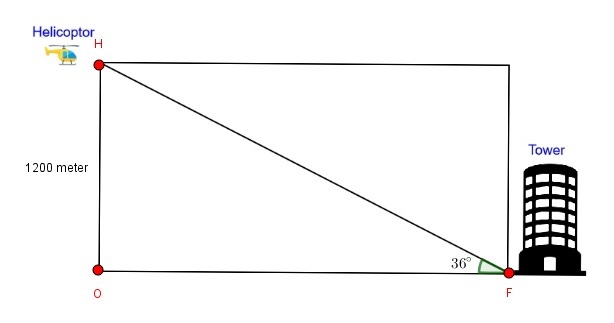
A helicopter is flying at an altitude of 1200°m. The angle of elevation from the tower on the ground to the plane measures 36°. How far is the building to the plane?
2 Answers
Explanation:

Given
To find
ABC is a right triangle with AC the hypotenuse. Applying Pythagoras theorem,
Please read the explanation.
Explanation:
Assumptions:
-
Angle of elevation from the tower on the ground to the plane:
#rArr# angle of elevation is from the foot of the tower#/_OFH=36^@# . -
How far is the building to the plane?:
#rArr# horizontal distance from the foot of the tower#bar (OF)# .
Please refer to the diagram (NOT drawn to scale) demonstrating the assumptions used:
We must find the magnitude of
For the
Since we must find the magnitude of
Using the calculator,
Hence,
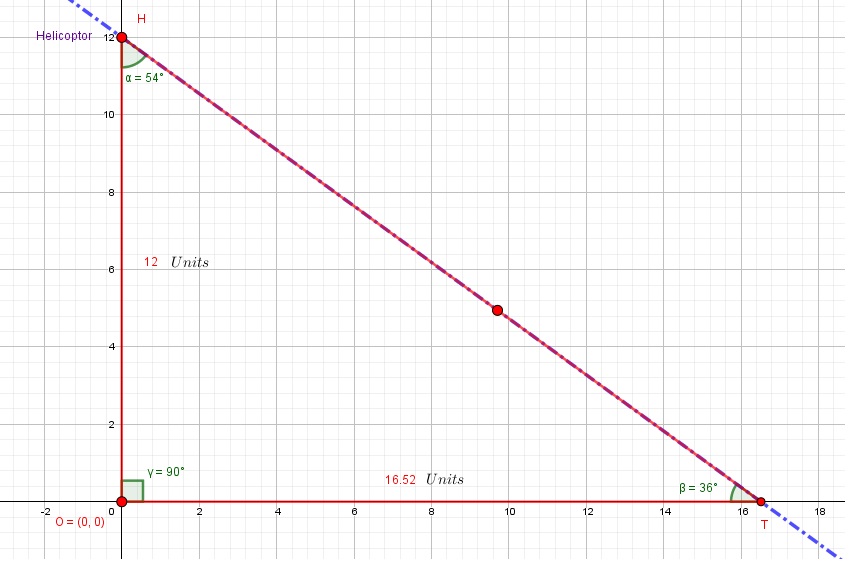
I have used a software to construct the problem for visual verification:
Scale Factor : 1 unit on the graph = 100 meters
All calculations are carried out using available functions in the software.