Question

A line segment is bisected by a line with the equation `- 2 y - x = 1`. If one end of the line segment is at `( 8 , 3 )`, where is the other end?

Answer 1

`-2y-x=1` bisects line segments between `(8,3)` and any point on the line `-2y-x=16`

Explanation:

Consider a horizontal line segment from `(8,3)` extending to the left
with a total length twice that of the horizontal distance from `(8,3)` to the line `-2y-x=1`.
From the diagram below we can see that this horizontal line will intersect `-2y-x=1` at `(-7,3)`
and will have its left-most end at `(-22,3)`

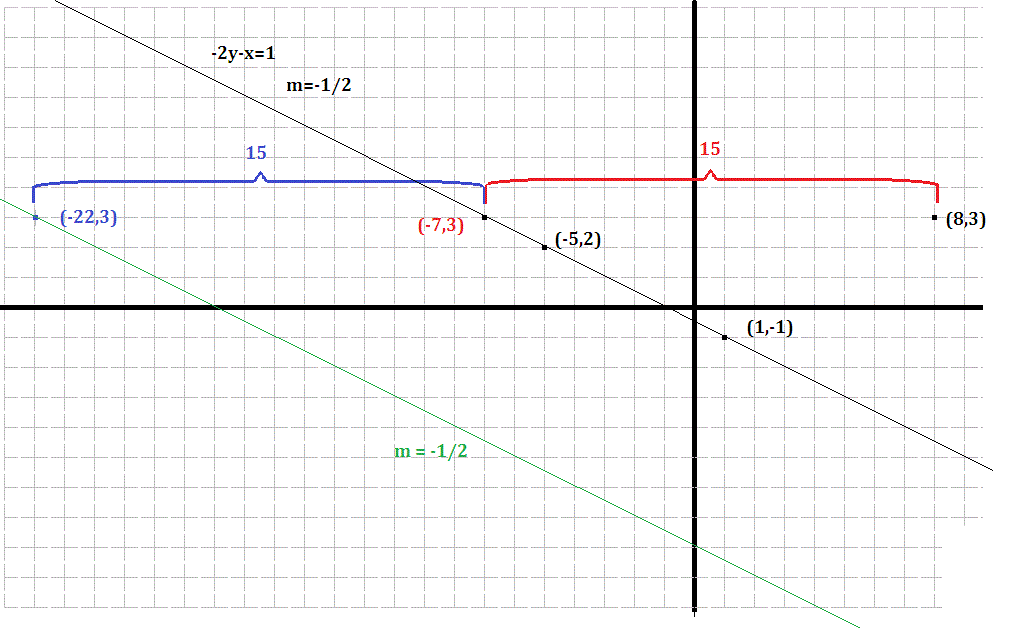
Obviously this horizontal line is bisected by `-2y-x=1`

Consider a line through this left-most point with the same slope as `-2y-x=1`.
Using the slope-point form we can see that the equation of this line can be written as `-2y-x=16`

Assertion
for any point `(x',y')` on this new line, the line segment between `(x',y')` and `(8,3)` will be bisected by `-2y-x=1`

This Assertion follows immediately from considering a modified version of the above diagram.

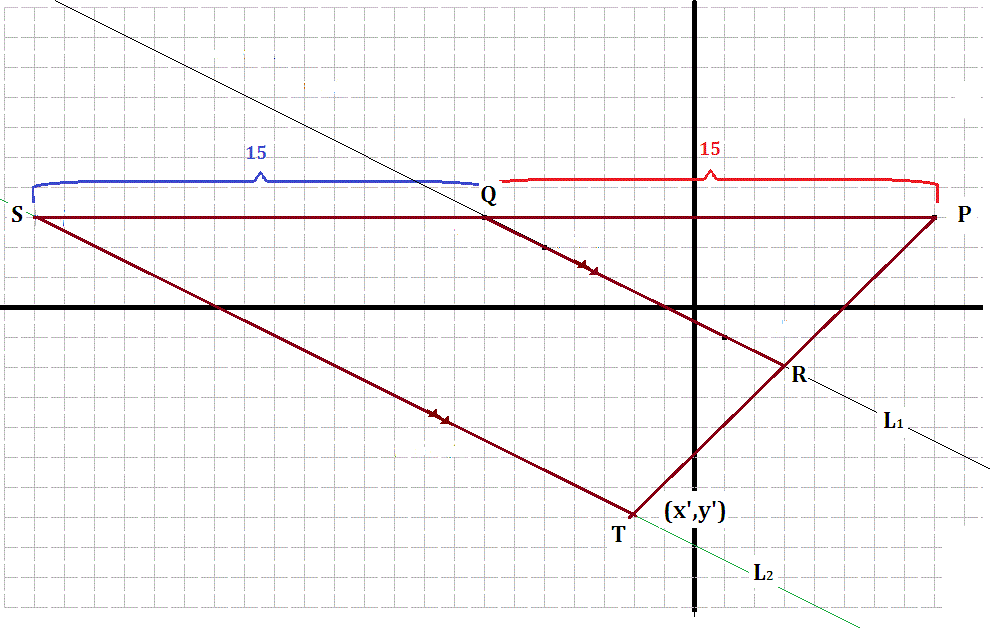
Since `QR||ST`
`color(white)("XXX")trianglePQR ~ trianglePST`

`color(white)("XXX")rArr PR:RT = PQ:QS = 1:1`

`rArr QR` bisects `PT` for any point `T=(x',y')` on `-2y-x=16`