A line segment is bisected by a line with the equation # 9 y + x = 5 #. If one end of the line segment is at #( 7 , 4 )#, where is the other end?
1 Answer
Jun 15, 2017
The other end is:
Here is a graph:
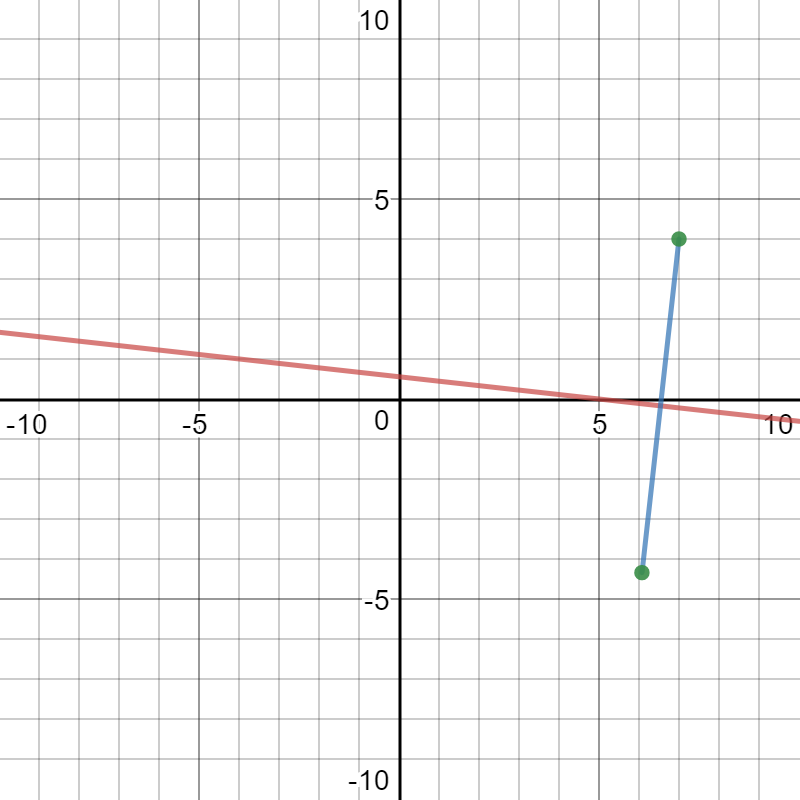
Explanation:
Given:
The family of perpendicular lines is:
Use the point
The equation of the bisected line is:
Multiply equation [2] by 9 and add to equation [1]:
Use the midpoint equation to find
Find
