Question

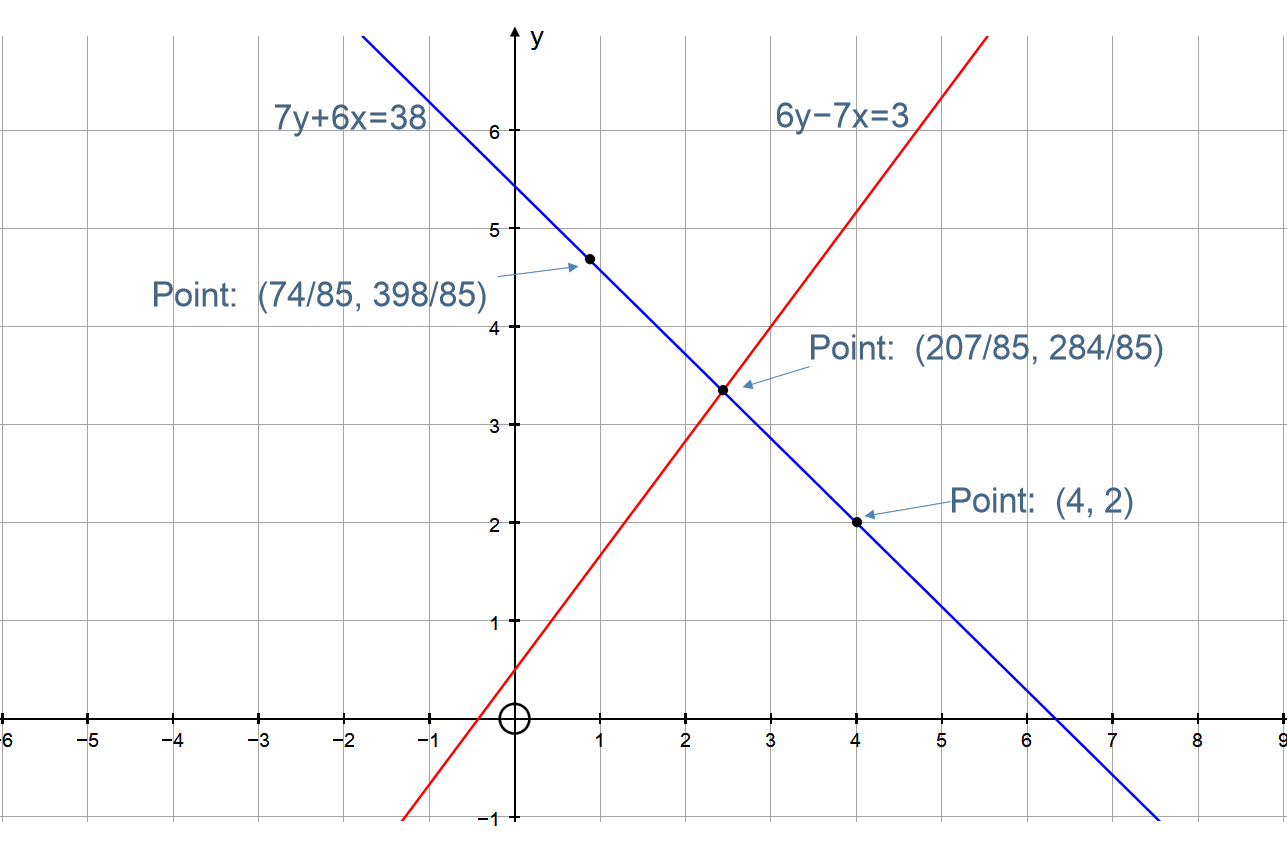
A line segment is bisected by line with the equation `6 y - 7 x = 3`. If one end of the line segment is at `(4 ,2 )`, where is the other end?

Answer 1

`color(blue)((74/85,398/85)`

Explanation:

First we note that if two lines are perpendicular then the product of their gradients is `-1`

We need to find the equation of a line that contains the point `(4,2)` and is perpendicular to `6y-7x=3`

Rearranging `\ \ \ \6y-7x=3`

`y=7/6x+1/2 \ \ \ \[1]`

If the gradient for our given line be `m`, then:

`m*7/6=-1=>m=-6/7`

Using point slope form of a line:

`y-2=-6/7(x-4)`

`y=-6/7x+38/7 \ \ \ \[2]`

Finding the point of intersection.

Solve `[1]` and `[2]` simultaneously:

`-6/7x+38/7-7/6x-1/2=0`

`x=207/85`

Substituting in `[1]`

`7/6(207/85)+1/2=284/85`

`(207/85,284/85)` are the coordinates of the midpoint:

The coordinates for the midpoint of a line is given by:

`((x_1+x_2)/2,(y_1+y_2)/2)`

Therefore:

`((4+x_2)/2,(2+y_2)/2)->(207/85,284/85)`

Hence:

`(4+x)/2=207/85=>x=74/85`

`(2+y)/2=284/85=>y=398/85`

Coordinates of the other end are:

`(74/85,398/85)`

PLOT: