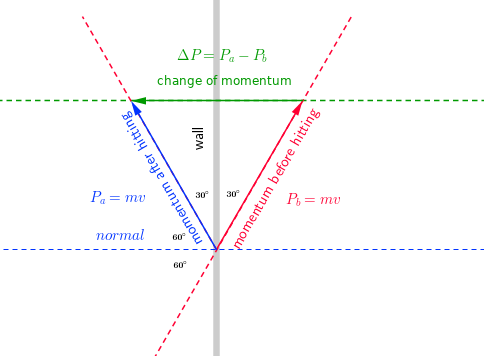
A particle of mass m strikes a wall at an angle of incidence 60° with velocity V elastically. Then the change in momentum is?

Question

12743 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-02-05T21:21:01

#"the cange in momentum :"Delta P= m v#

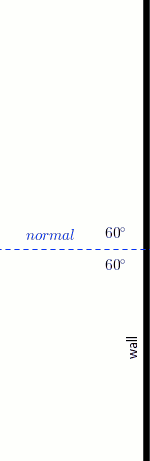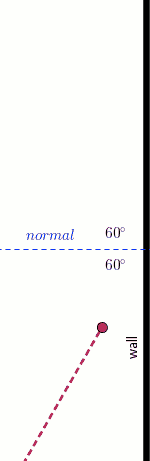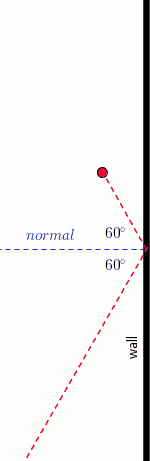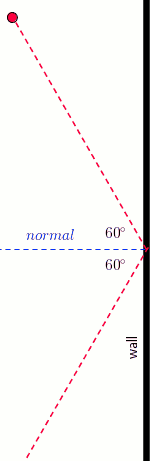
enter image source here

The reflection of the particle on the wall is shown as animation.
Reflection of the particle will be comply to the Snell's laws.
-Collision is perfectly elastic so energy does not lost.
The magnitude of the velocity at the end of the collision does not change.
You can find momentum change in different ways.
The geometric calculation is shown below.

enter image source here

#Delta vec P=vec P_a-vec P_b#

#vec P_b=m vec v#

#vec P_a=m vec v#

#Delta P=sqrt( P_b^2+ P_a^2-2 P_b P_a cos theta)#

#Delta P=sqrt((m v)^2+(m v)^2-2.m v. m v* cos (60))#

#cos (60)=1/2#

#Delta P=sqrt((m v)^2+(m v)^2-cancel(2). m v. m v* 1/cancel(2))#

#Delta P=sqrt((m v)^2+cancel((m v)^2)-cancel((m v)^2))#

#Delta P=sqrt((m v)^2)#

#Delta P= m v#