Question

A placement exam for entrance into a math class yields a mean of 80 and a standard deviation of 10. How do you find the percentage of scores that lie between 60 and 80?

The distribution of the scores is roughly bell shaped. Use the empirical rule.

Answer 1

The percentage of scores that lie between `60` and `80` is `47.72%`.

Explanation:

Let us assume that distribution is normal. As mean is `80` and standard deviation is `10`,

`z-score for`60`is`(60-80)/10=-2# and

`z-score for`80`is`(80-80)/10=0#

On a normal curve, it should appear as follows:

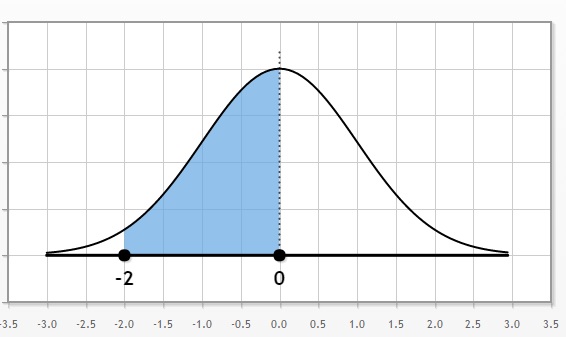
the charts/ tables show the area under the curve between `z=0` and `z=p`, where `p` is the `z`-score and as normal curve is symmetric, sign of `z` does not matter.

Hence, the area under the curve for `z`-score `-2` is `0.4772`, which means the area under normal curve from `z=+2` to `z=0`
(or `z=0` to `z=-2`).

Hence, the percentage of scores that lie between `60` and `80` is `47.72%`.