A projectile is shot from the ground at an angle of #(5 pi)/12 # and a speed of #6/5 m/s#. Factoring in both horizontal and vertical movement, what will the projectile's distance from the starting point be when it reaches its maximum height?
1 Answer
Explanation:
I copied, pasted and changed the values of this answer:
https://socratic.org/questions/a-projectile-is-shot-from-the-ground-at-an-angle-of-pi-6-and-a-speed-of-5-m-s-wh#425323
If you have difficulties understanding projectiles motion visit this:
https://socratic.org/physics/2d-motion/projectile-motion
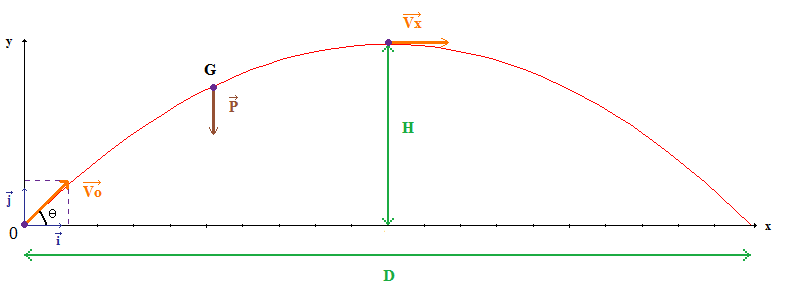
We have to calculate the initial speed in x and in y:
Let's calculate the distance in y (height):
We have to know when is the projectile at its maximum height. Let's use the equation of the speed for an object with acceleration:
The acceleration is
To find the distance in y we have to use the equation of distance:
To find the distance in x we have to use another time the equation of distance (this time there's no acceleration):

