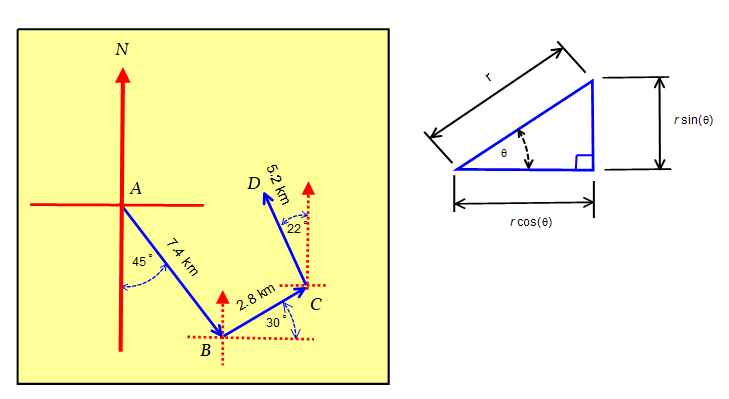
Often a good idea to draw a very quick and rough sketch to give you an idea of what you are having to deal with.
Set #rarr# as positive thus #larr# is negative
Set #uarr# as positive thus #darr# is negative
#color(brown)("Consider the horizontal")#
#AB_h=+[7.4xxsin(45^o)]~~+5.23159..#
#BC_h=+[2.8xxcos(30^o)]~~+2.42487..#
#CD_h=-[5.2xxsin(22^o)]~~-1.94795..#
Sum #~~+5.70950....#
#color(brown)("Consider the vertical")#
#AB_h=+[7.4xxcos(45^o)]~~-5.23259.....#
#BC_h=+[2.8xxsin(30^o)]=+1.4" "...#
#CD_h=-[5.2xxcos(22^o)]~~+4.82135...#
Sum #~~+0.98876....#
So we end up with:
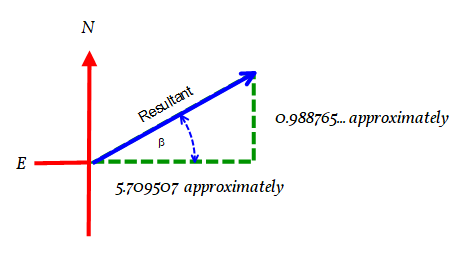
#beta =tan^(-1)(0.988765...)/(5.709507..)~~9.82497..."degrees East North"#
#"Resultant "r ~~ sqrt((0.988765..)^2+(5.709507..)^2)#
#r=5.794491212bar(12)#
~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~~
#color(blue)("This is a repeating decimal so a rational number")#
#color(brown)("Converting to an exact fractional answer")#
Set #x_1=5.794491212bar(12)#
Set#x_2=0.794491212bar(12) #
#10000000x_2=7944912.1212bar(12)#
#ul(color(white)("d0")100000x_2=color(white)("00")79449.1212bar(12)larr" Subtract")#
#color(white)("d")9900000x_2=7865463#
#x_2=7865463/9900000#
#r=x_1 = 5 color(white)("d")7865463/9900000 color(red)(larr" What an awful number!!!")#
#color(red)("I'm sticking with the decimal!!")#



