A stream 50m wide is flowing at 15m/s. Isaac can swim at a speed of 20m/s in calm water. In what direction ( angle ) must Isaac set off if he must reach the opposite bank by the shortest route?
1 Answer
Mar 1, 2018
See the diagram,it explains what the situation is going to be for achieving the purpose,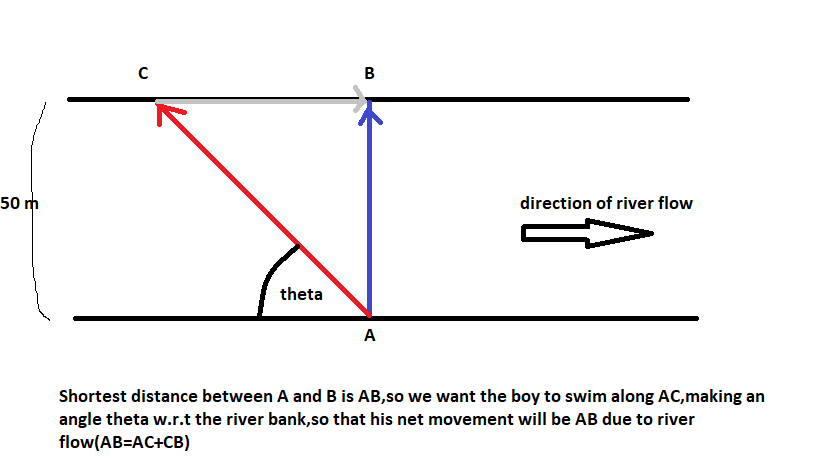
So,let he starts swimmimg at an angle
So,we get,
or,
So,
So,he will move from
So,time taken to reach

