A triangle has corners at #(3,7)#, #(4,1)#, and #(8,2)#. What are the endpoints and lengths of the triangle's perpendicular bisectors?
1 Answer
- End points of the perpendiclar bisectors
- Lengths of the perpendicular bisectors are
Explanation:
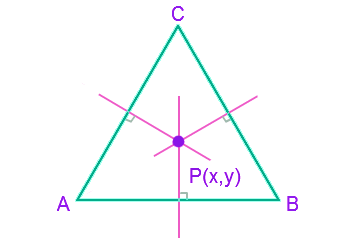
A, B, C are the vertices and Let D, E, F be the mid points of the sides a, b, c respectively.
Midpoint of BC = D = (x2 + x1)/2, (y2 + y1)/2 = (4+8)/2, (1+2)/2 =
(6,3/2)#
Slope of BC
Slope of the perpendicular bisector through D =
Equation of perpendicular bisector passing through mid point D using standard form of equation
Similarly,
Mid point of CA =
Slope of CA = m_(CA) = (2-7)/(8-3) = -1#
Slope of the perpendicular bisector through E =
Equation of perpendicular bisector passing through mid point E is
Similarly,
Mid point of AB =
Slope of AB= m_(AB) = (1-7)/(4-3) = -6#
Slope of the perpendicular bisector through F =
Equation of perpendicular bisector passing through mid point F is
Solving Eqns (D), (E), we get the coordinates of circumcenter P.
This can be verified by solving Eqns (E), (F).
and the answer is
Length of the perpendicular bisectors PD
Length of perpendicular bisector PE
Length of perpendicular bisector PF
7/2, 4
