A truck pulls boxes up an incline plane. The truck can exert a maximum force of 4,500 N4,500N. If the plane's incline is pi/8 π8 and the coefficient of friction is 4/3 43, what is the maximum mass that can be pulled up at one time?
1 Answer
Jan 15, 2018
The mass is
Explanation:
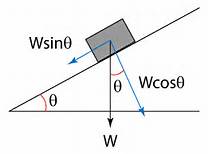
Resolving in the direction parallel to the plane
Let the force exerted by the truck be
Let the frictional force be
The coefficient of friction
The normal force is
The angle of the plane is
The acceleration due to gravity is
Therefore,

