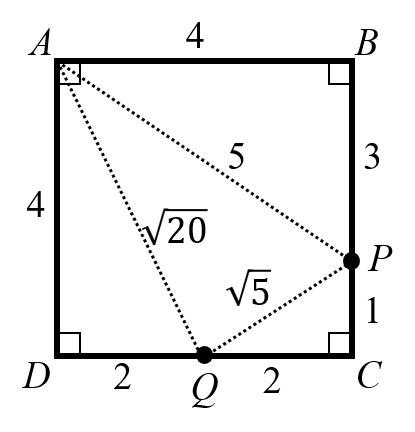
Assume that the length of a side of square ABCD is 4 units. Then
#BP=3#
#PC=1#
#QC=2#
#DQ=2#
#AD=4#
#AB=4#
Consider the right triangle #PQC# where angle #C# is 90 degrees. #PC# and #QC# are the legs of the right triangle. Since #PC=1# and #QC=2#, by the Pythagorean Theorem, the length of the hypotenuse #PQ=sqrt(1^2+2^2)=sqrt(5)#.
Now consider the right triangle #ADQ# where angle #D# is 90 degrees. #AD# and #DQ# are the legs of this triangle. Since #AD=4# and #DQ=2#, by the Pythagorean Theorem, the length of the hypotenuse #AQ=sqrt(4^2+2^2)=sqrt(20)#.
So
#(AQ)/(PQ)=sqrt(20)/sqrt(5)=sqrt(4)=2#.
Therefore
#AQ=2PQ#.
Now consider right triangle #ABP# where angle #B# is 90 degrees. #AB# and #BP# are the legs of of the right triangle. Since #AB=4# and #BP=3#, the length of the hypotenuse #AP=sqrt(4^2+3^2)=5#.
Now consider triangle #APQ#. Two of its sides are of length #sqrt(5)# and #sqrt(20)#. Note that if we add squares the length of both of these sides, we get the square of length of the third side
#(sqrt(5))^2+(sqrt(20))^2=5^2#
which means that triangle #APQ# must be a right triangle. We have already proven that the ratio of the length of its longer leg to its shorter leg is 2. The same is true for right triangle #CPQ#, so #APQ# and #CPQ# must be similar (by the S ide- A ngle- S ide Theorem).


