An object with a mass of #6 kg# is on a plane with an incline of #pi/12 #. If the object is being pushed up the plane with # 6 N # of force, what is the net force on the object?
1 Answer
Explanation:
I'll assume the surface is frictionless, as there is no information regarding friction given.
Referring to the image below:
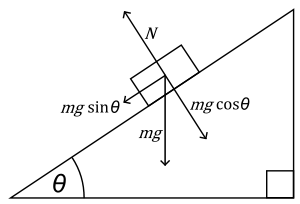
I'll call the positive
We know the normal force
Since the surface is (supposedly) frictionless, the only forces being applied are
-
gravitation force, equal to
#mgsintheta# (directed down the incline) -
applied force, equal to
#6# #"N"# (directed up the incline)
We need to find the quantity
-
#m = 6# #"kg"# -
#g = 9.81# #"m/s"^2# -
#theta = pi/12#
Therefore,
The net horizontal force
directed down the plane
