Another Circle Theorem?

enter image source here

1 Answer
Tom M.
Jan 10, 2018

Proof detailed below

Explanation:

I've taken your diagram throughout (thank you!)

We're going to give some names to parts of your diagram:

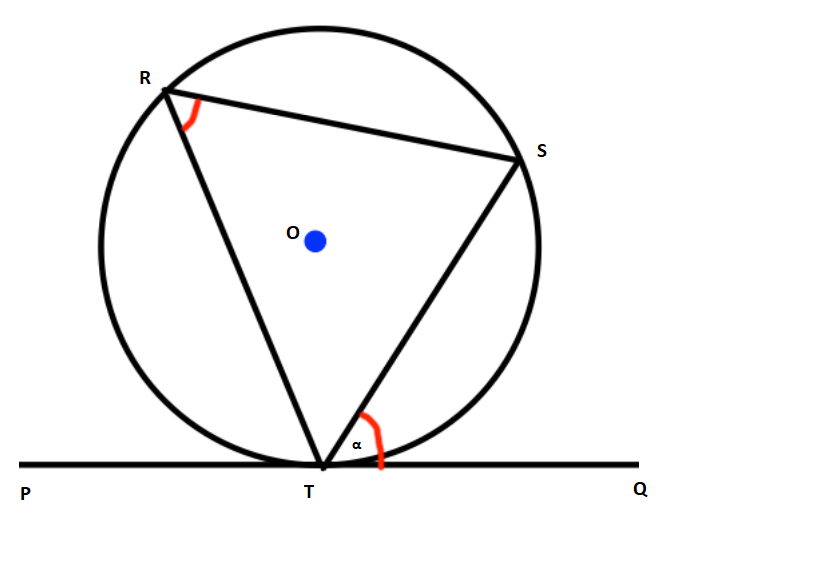
enter image source here
Let #/_STQ=alpha#

We will construct two radii between #OT# and #OS#

enter image source here
Since the angle between a tangent and a radius is a right angle, #/_OTP=90^@#

#:. /_OTS=90^@-alpha#

Since OT and OS are both radii:

#OS=OT#

#:. triangleOST" is isosceles"#
#:./_OST=/_OTS#
#=90^@-alpha#

Since there are 180 degrees in a triangle;
#90-alpha+90-alpha+/_SOT=180#
#180-2alpha+/_SOT=180#
#-2alpha+/_SOT=0#
#/_SOT=2alpha#

Final diagram to make the last step clear:
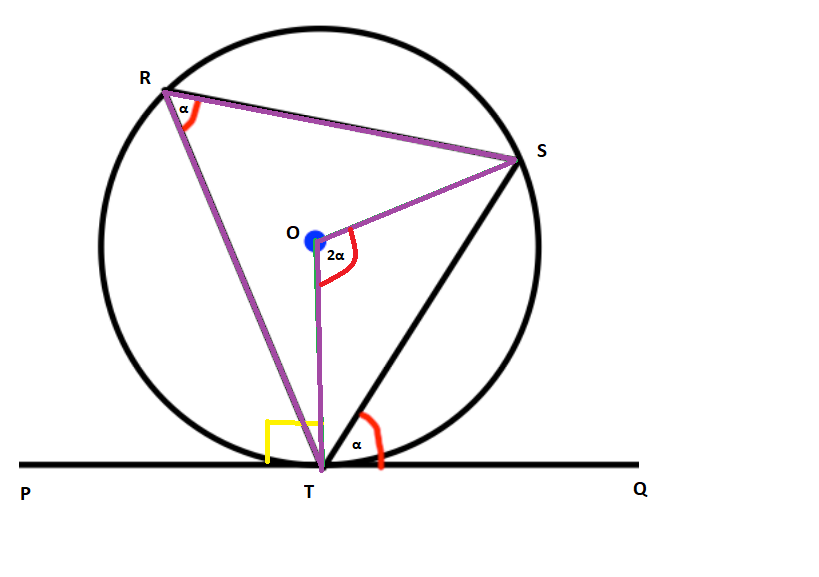
enter image source here
I have used purple lines to make this last bit clear. We will use another circle theorem:
The angle at the centre is twice that at the circumference

#:./_SOT=2/_TRS#
#2alpha=2/_TRS#
#/_TRS=alpha=/_STQ#

#/_TRS=/_STQ :.#
the angle between a tangent and a chord at the point of contact is equal to the angle in the alternate segment

Related questions
Impact of this question
1732 views around the world
You can reuse this answer
Creative Commons License