Question

Buffer Solution Help ?????

Answer 1

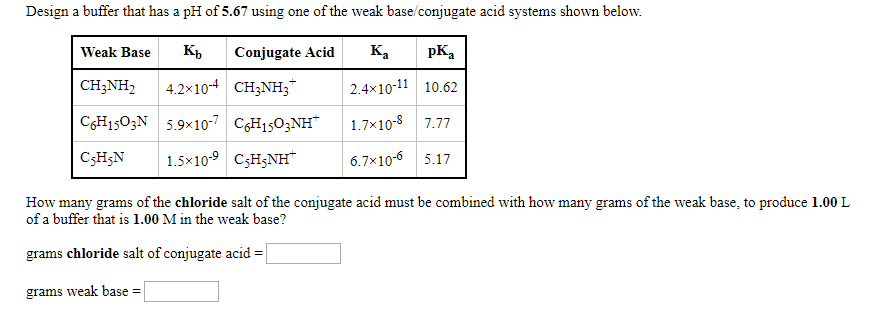
We must dissolve 78.1 g of the weak base (pyridine) and 69.5 g of pyridinium chloride in enough water to make 1 L of solution.

Explanation:

The best buffer is one in which `"p"K_text(a)` close to the desired buffer pH.

The acid with the closest `"p"K_text(a)` is the pyridinium ion, `"C"_5"H"_5"NH"^"+"`.

The chemical equation for the equilibrium is

`"C"_5"H"_5"NH"^"+" + "H"_2"O" ⇌ "C"_5"H"_5"N" + "H"_3"O"^"+""`

Let's rewrite this equation as

`"BH"^"+" + "H"_2"O" ⇌ "B" + "H"_3"O"^"+"`

We can apply the Henderson-Hasselbalch equation:

`color(blue)(bar(ul(|color(white)(a/a)"pH" = "p"K_text(a) + log((["B"])/(["BH"^"+"]))color(white)(a/a)|)))" "`

`5.67 = 5.17 + log((["B"])/(["BH"^"+"]))"`

`log((["B"])/(["BH"^"+"]))" = 5.67 - 5.17 = 0.50`

`(["B"])/(["BH"^"+"])" = e^0.50 = 1.65`

`["BH"^"+"] = ["B"]/1.65 = "1.00 mol/L"/1.65 = "0.607 mol/L"`

So, we want 1 L of a buffer that contains 1.00 mol of `"B"color(white)(l)("C"_5"H"_5"N")` and 0.607 mol of `"BH"^"+""Cl"^"-" ("C"_5"H"_5"NCl")`.

`"Mass of C"_5"H"_5"N" = 1.00 color(red)(cancel(color(black)("mol C"_5"H"_5"N"))) × ("78.10 g C"_5"H"_5"N")/(1 color(red)(cancel(color(black)("mol C"_5"H"_5"N")))) = "78.1 g C"_5"H"_5"N"`

`"Mass of C"_5"H"_5"NCl" = 0.607 color(red)(cancel(color(black)("mol C"_5"H"_5"NCl"))) × ("114.55 g C"_5"H"_5"NCl")/(1 color(red)(cancel(color(black)("mol C"_5"H"_5"NCl")))) = "69.5 g C"_5"H"_5"NCl"`