Calculate activity of a radioactive substance after 3 hours ?
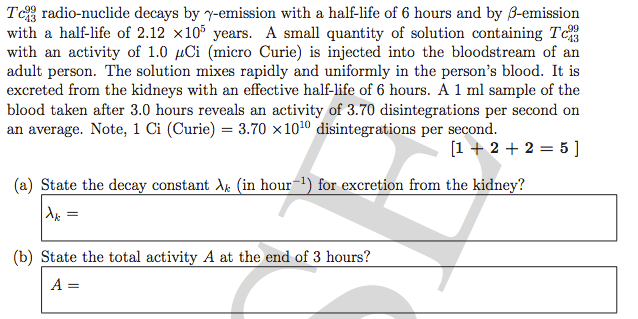
Please help me solve this , The 1ml Blood part of the question is for some further parts and not related to part (a) and (b).

Please help me solve this , The 1ml Blood part of the question is for some further parts and not related to part (a) and (b).
1 Answer
This is what I get
Explanation:
For decay process of an unstable nucleus is entirely random. It is impossible to predict when a specific atom will decay. However, we talk about probability of decay of a particular nucleus at a given instant in time.
Therefore, in a given sample of radioactive material, the number of decay events
#- (d N) /(d t)prop N#
# (d N) /(d t)-=A=lambda N# ........(1)
where#A# is activity and#lambda# is proportionality constant also called decay constant.
Alternatively the probability of decay
#=>- (d N) /Nprop dt#
#=>- (d N)/ N = λ d t # .....(2)
The negative sign indicates that#N# decreases with increase of time.
This first-order differential equation has a solution of the form
#N ( t ) = N_0\ e ^ (-λt)# .......(3)
where#N_0# is the value of#N# at time#t = 0# .
Here we introduce term half life
#N(t)=N_0(1/2)^(t/t_(1/2))# .......(4)
Comparing (3) and (4) we get
#(1/2)^(t/t_(1/2))=e ^ (-λt)#
Tanking natural logarithm of both sides
#ln(1/2)^(t/t_(1/2))=lne ^ (-λt)#
#=>(t/t_(1/2))ln(1/2)= (-λt)lne #
#=>t_(1/2)=ln2/lambda # .........(5)
It is given that sample decays via two exponential decay processes simultaneously. Therefore, the actual half-life
#1/ T_(1 / 2) = 1/ t_1 + 1/ t_2# ..........(6)
Two half lives are given as
(a) Inserting given value in (5)
#6=ln2/lambda_k #
#=>lambda_k=ln2/6 #
#=>lambda_k=0.1155\ hr^-1#
(b) Initial activity is given
From (3) number of nuclei remaining after
#N(3\ hr)=N_0\ e ^ (-0.1155xx3)#
#N(3\ hr)=0.707N_0#
From (1)
#A(3\ hr)=0.707A_0#
#A(3\ hr)=0.707\ muCi#
.-.-.-.-.-.-.-.-.-.-.-
Specifically
Inserting various values in (1) we get
#1.0xx10^-6xx(3.70xx10^10)=0.1155 N_0#
#=> N_0=(1.0xx10^-6)/0.1155xx(3.70xx10^10)#
