Calculate the height from the Earth's surface that a satellite must attain in order to be in geosynchronous orbit and the satellite's velocity?
A geosynchronous orbit is one in which the satellite remains "in place" above the same spot on the Earth's surface.
Radius of the Earth- #6.38 xx 10^6# m
Gravitation Constant- #6.67 xx 10^(-11)m^3kg^(-1)s^(-2)#
Mass of the Earth- # 5.97xx 10^24# kg
A geosynchronous orbit is one in which the satellite remains "in place" above the same spot on the Earth's surface.
Radius of the Earth-
Gravitation Constant-
Mass of the Earth-
2 Answers
Explanation:
Geosynchronous means that the satellite has same period as the earth, back to the same place in 24 hours.
T =24hrs = 86400 s
And let
h = height of the satellite from the surface of the earth.
r = radius of the satellite from the center of the Earth
The gravitational pull from the earth causes the satellite to go in orbit (otherwise it flies away. Hence the gravity is the cause of the centripetal force
Because the orbital speed
Substitute the proper values to compute h. The key is to recognize T is 24 hours.
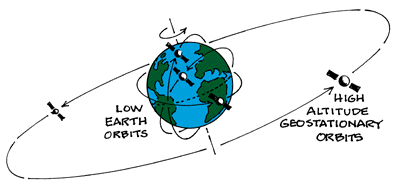
A geosynchronous orbit is a circular orbit that lies on the Earth's equatorial plane and follow the direction of the Earth's rotation in the period equal to the Earth's rotational period (one sidereal day) and thus it appears motionless, at a fixed position in the sky, to ground observers.
Given
Earth's rotational period (one sidereal day)
Let the height of the orbit from Earth's surface be
Equating centripetal force with the gravitational force we can write
So the height from the Earth's surface that a satellite must attain in order to be in geosynchronous orbit
And the satellite's velocity


