Construct a regular pentagon using a compass and straight edge? Explain each step
1 Answer
Calculate the length of the radius of the circle for the chosen length of the sides of the pentagon.
Explanation:
You can use sides of any length you like, but for this example I have used the sides of the regular pentagon as 4cm.
If the sides of the pentagon are to be 4cm, then the circle must have a radius of 3.4cm
Draw a circle with a radius of 3.4cm and mark point A on the circumference.
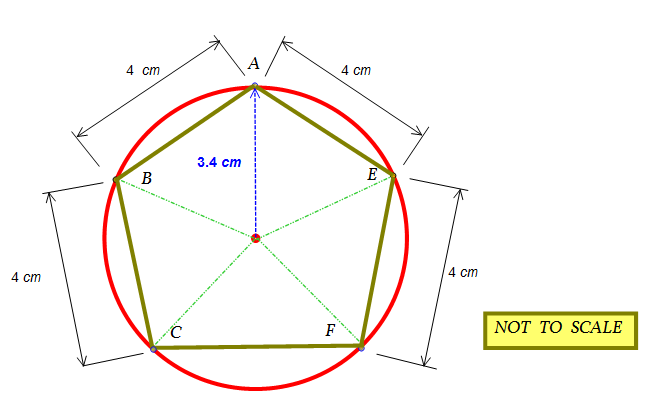
Thank you Tony B
With radius 4cm and centre A, mark two points B and E on the circumference.
With centre B and radius 4cm, mark C on the circumference.
With centre E and radius 4cm, mark F on the circumference.
As an accuracy check, an arc with Centre F and radius 4cm should coincide with C.
Why does this work?
A regular polygon will always fit into a circle, with the vertices on the circumference. The difficulty is in having the correct radius of the circle for the correct length of the side of the polygon. Once these are known it is a matter of marking off equidistant points on the circumference.
An isosceles triangle inside a pentagon will have base angles of 54°
The hypotenuse represents the required radius of the circle to be drawn and can be calculated using trig.
A hexagon is the only polygon made up of equilateral triangles, where the radius of the circle is the same as the sides of the hexagon.

