Determine the length AB in the figure below without using Cosine and Sine formulas?
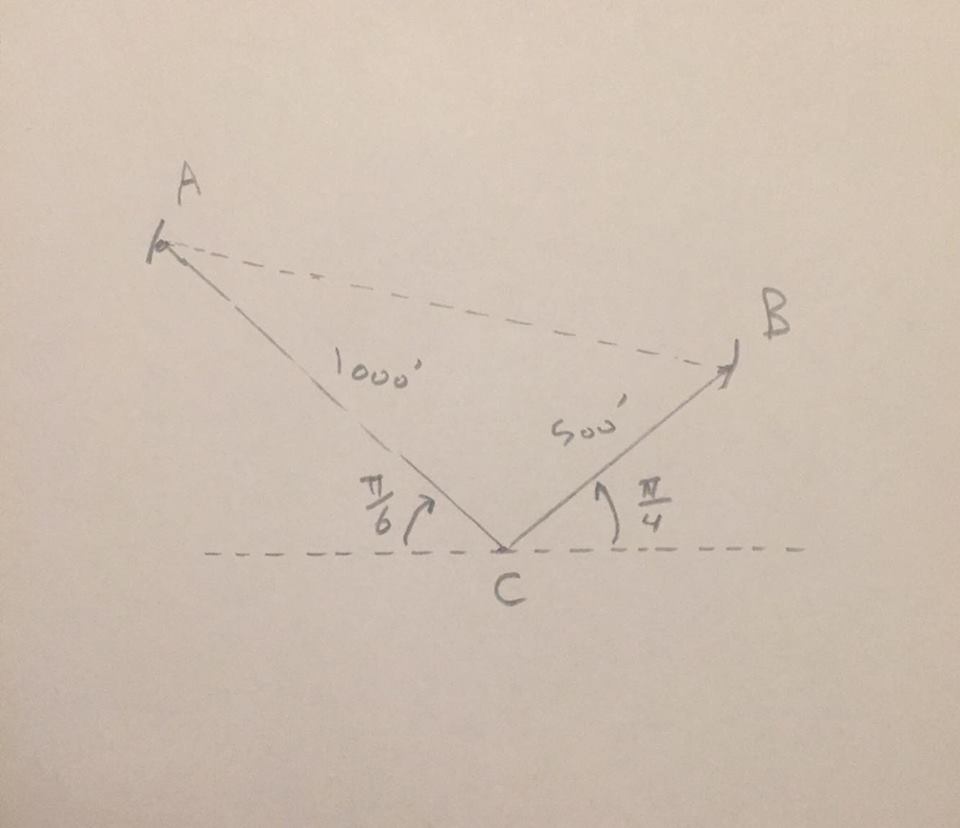
1 Answer
Feb 24, 2018
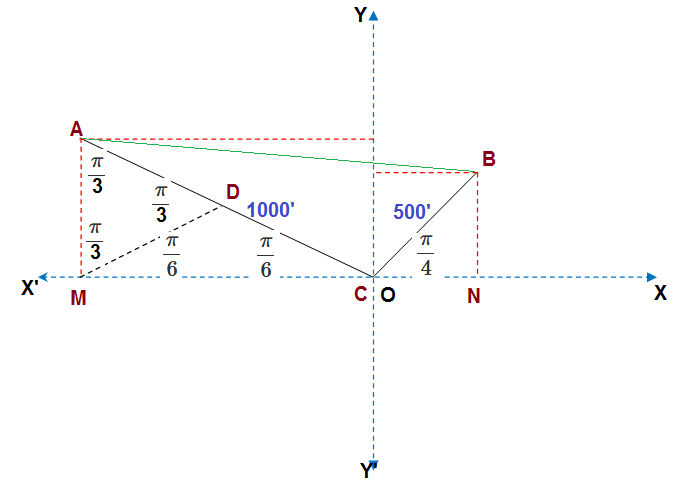
To determine the length of AB without using trigonometry let us imagine C as the origin and the given line through C as X-axis .
Perpendiculars AM and BN are drawn on X-axis .
Now
Now
So coordinates of
Now in
So
Hence
Hence coordinates of
So length of AB

