Do you know grade 9 math/a bit about similar triangles? Please help!!!
1 Answer
Nov 4, 2017
Explanation:
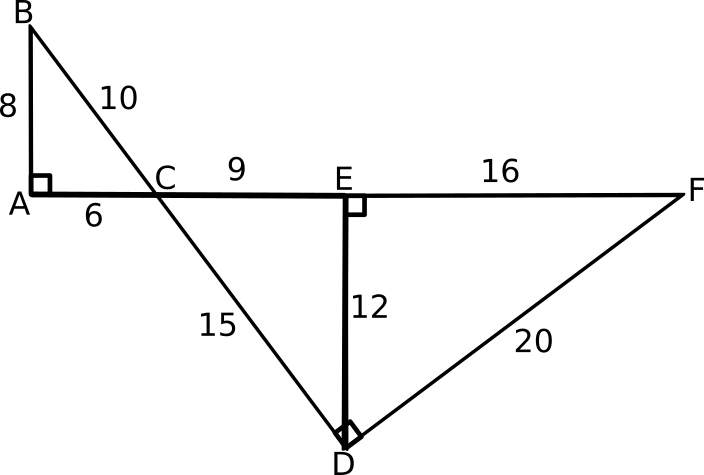
The diagram is definitely not drawn to scale!
Let us assume that we are looking for sides of integral length.
Looking at the side of length
Note that
Note that
#ED = 4/3*9 = 12#
#CD = 5/3*9 = 15#
Then
#EF = 4/5*20 = 16#
#AC = 3/4*8 = 6#
#BC = 5/4*8 = 10#
Note that:
#CF = CE+EF=9+16 = 25 = 5/4*20# as required.