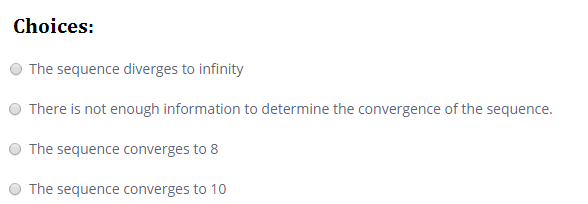
Does the sequence defined by #a_1=2#, #a_(n+1)=72/(1+a_n)# converge? What does it converge to?

Question

5663 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-09-25T02:59:13

See below.

If the sequence converges then

#a_(n+1)= a_oo = 72/(1+a_n)=72/(1+a_oo)#

or

#a_oo = 72/(1+a_oo)# and solving for #a_oo# we get at

#a_oo = {-9,8}#

Now analyzing the behavior of the transformation

#f(x)=72/(1+x)# we have

#f'(x) = -72/(1+x)^2# and

#abs(f'(8)) = 8/9 < 1 rArr 8# is a stable sequence limit point
#abs(f'(-9)) = 9/8 > 1 rArr -9# is an unstable sequence limit point.