Find an equation whose graph is a horizontally opening parabola that passes through (20, -3), (12,1), and (33,-2)?
1 Answer
The equation is:
Here is a graph of the parabola and the 3 points:
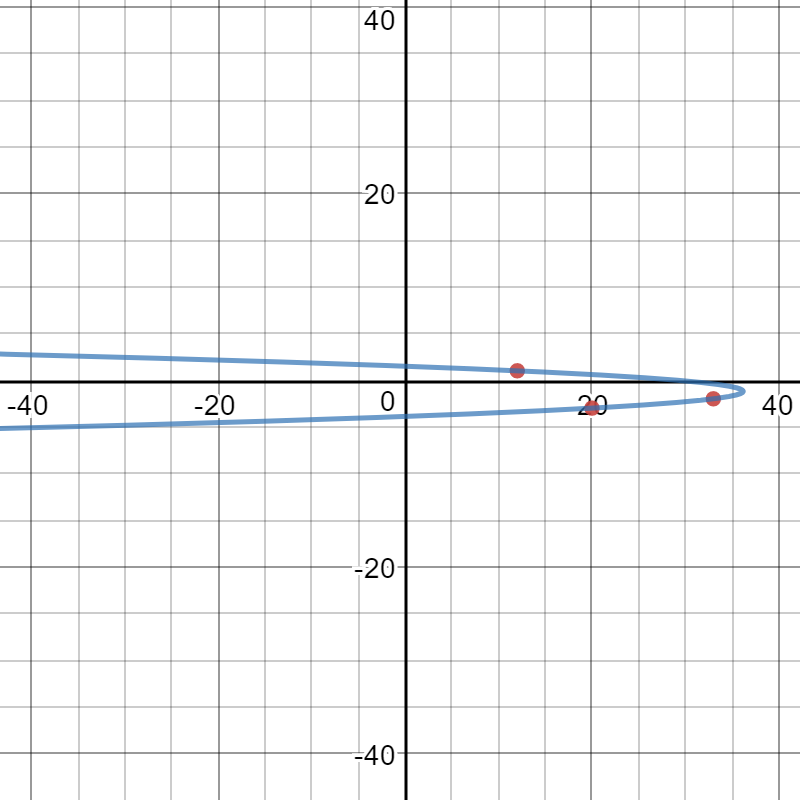
Explanation:
The standard form for a horizontally opening parabola is:
Substituting the point
This equation translates into the first row of an augmented matrix as:
Substituting the point
This equation translates into the second row of the augmented matrix as:
Substituting the point
This equation translates into the second row of the augmented matrix as:
Perform elementary row operations, until an identity matrix is obtained on the left:
We have an identity matrix on the left, therefore, we can read the solutions on the right,
The equation is:
