Find the coordinate of the point at which the perpendicular bisector of the line joining (2,7) to (10,3) meet the x- axis please solve through making the diagram of line point etc?

Question

11370 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2018-04-30T14:22:42

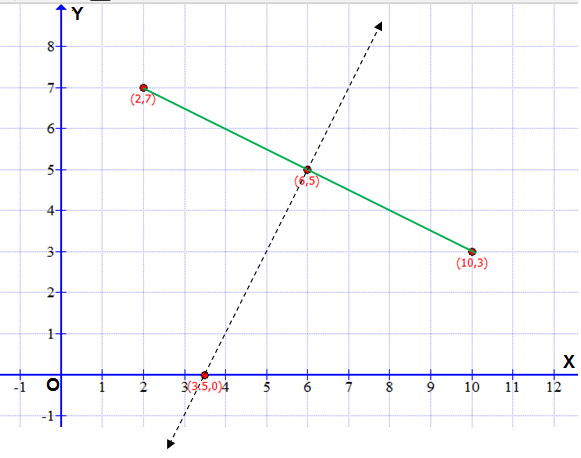
enter image source here

The coordinates of the mid point of the line joining (2,7) to (10,3) are #((2+10)/2,(7+3)/2)equiv(6,5)#

Let the coordinates of the point of intersection of the perpendicular bisector of the line segment joining the two given point be #(h,0)#.

So the gradient of the perpendicular bisector #(5-0)/(6-h)=5/(6-h)#

Again the slope of the line segment is #=(7-3)/(2-10)=-1/2#

As the bisector is perpendicular to the line segment then

#5/(6-h)xx(-1/2)=-1#

#=>5=12-2h#

#=>h=7/2=3.5#

So the coordinates of the point of intersection of the perpendicular bisector with the X-axis is #(3.5,0)#