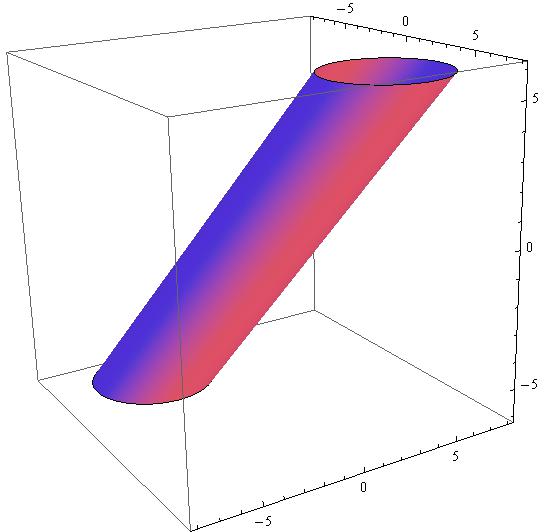
Find the equation of the cylinder whose base is circle #x^2+y^2=9, z=0# and the axis is #x/4=y/3=z/5#?

Question

2554 views around the world

You can reuse this answer
Creative Commons License

Answer 1 · 2017-07-11T11:55:58

See below

Given the line

#(x-x_0)/alpha=(y-y_0)/beta=(z-z_0)/gamma# with

#x_0^2+y_0^2-9=0# which is the basis, with #z_0=0#

and substituting

#x_0 = x-alpha/gamma z#
#y_0= y-beta/gamma z# we obtain

# (x - (4 z)/5)^2 + (y - (3 z)/5)^2-9=0# which is the cylinder equation.

Attached a plot showing the cylindrical surface

enter image source here