Question

Find the volume of the region bounded by y=sqrt(z-x^2) and x^2+y^2+2z=12?

find the volume of the region bounded by y=sqrt(z-x^2) and x^2+y^2+2z=12?

Answer 1

`=12 pi`

Explanation:

this is symmetric about z axis so we use polar co-ords

first paraboloid

`y=sqrt(z-x^2)`
`y^2=z-x^2`
`z_1 = y^2 + x^2 = r^2`

second

`x^2+y^2+2z=12`
`z_2=(12 - (x^2+y^2))/2 = 6 - r^2/2`

`z_1 = z_2 implies 6 - r^2/2 = r^2`
`implies r = 2`
(and `z_1 = z_2 = 4`)

here is a 2-D plot:

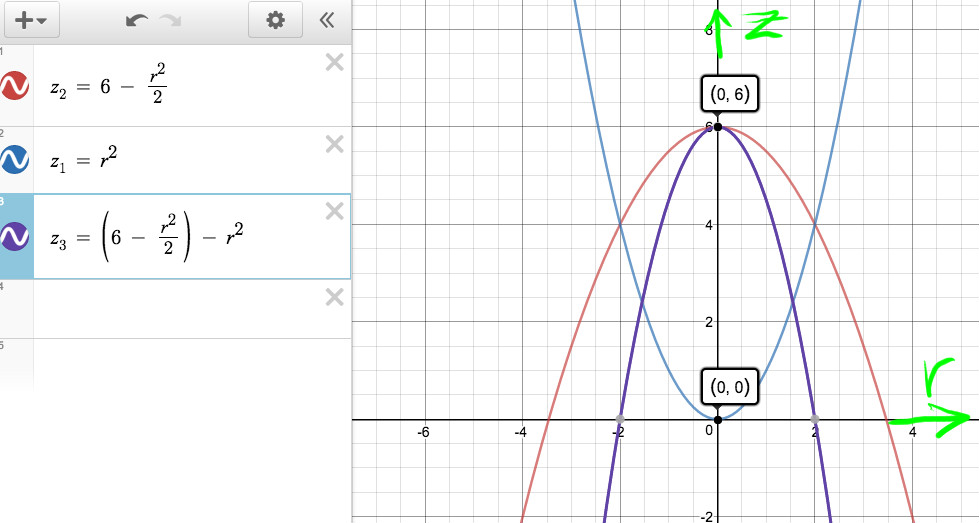
We need to find the volume that is made by revolving the area between the red and blue curves around the z-axis. That is the area under the purple curve, which is entered as `z_3 = z_2 - z_1` in the box on the left.

Ie, we need to find volume

`V = int_A \ (z_2 - z_1) dA`

where volume element in polar is `dA = r \ dr \ d theta`

`implies int_0^(2 pi) int_0^2 (6 - 3/2 r^2) r \ dr \ d theta`

`= int_0^(2 pi) \ d theta * \ int_0^2 6r - 3/2 r^3 \ dr`

`= 2 pi [ 3r^2 - 3/8 r^4 ]_0^2`

`= 2 pi [ 3r^2( 1 - 1/8 r^2) ]_0^2`

`= 2 pi ( 12( 1 - 1/2) )`

`=12 pi`

As an aside, @abubakar wanted this expressed as a triple integral . I originally skipped that step because it just adds extra notation but if we start with the general volume element and switching straight to cylindrical co-ordinates, we can say that

`int_V dV = int_(theta_0)^(theta_1) \ int_(r_0)^(r_1) \ int_(z_0)^(z_1) \ r dr d theta dz`

now neither r or z depend upon `theta` so we can immediately, using Fubini's theorem, lift `theta` outside the integration as it is independent so we have

`int_V dV =( int_(0)^(2 pi) d theta )*( \ int_(r_0)^(r_1) \ int_(z_0)^(z_1) \ r dr dz )`

`=2 pi \ int_(r_0)^(r_1) \ int_(z_0)^(z_1) \ r dr dz`

I did that to simplify the next bit, because we have `r = r(z)` and (z = z(r)#. z and r are inter-dependent. clearly!

In general terms, to do this we need either do

`=2 pi \ int_(z_0)^(z_1) \ int_(r = r_0(z))^(r_1(z)) \ r \ dr \ dz`

OR

`=2 pi \ int_(r_0)^(r_1) \ int_(z = z_0(r))^(z_1(r)) \ r \ dz \ dr`

The explanation and drawings here are helpful

The key to this is that these are iterated integrals. The `dA = dx \ dy = r \ dr \ d theta` element notation is very useful but perhaps misleading.

Answer 2

A graphic contribution.

Explanation:

A graphic contribution.