For what value of c, the lines which join the origin to the points of intersection of the given straight line and the curve be at right angle?
For what value of c, the lines which join the origin to the points of intersection of straight line #x-y+c=0# and the curve #x^2+y^2+4x-6y-36=0# be at right angle?
For what value of c, the lines which join the origin to the points of intersection of straight line
1 Answer
Explanation:
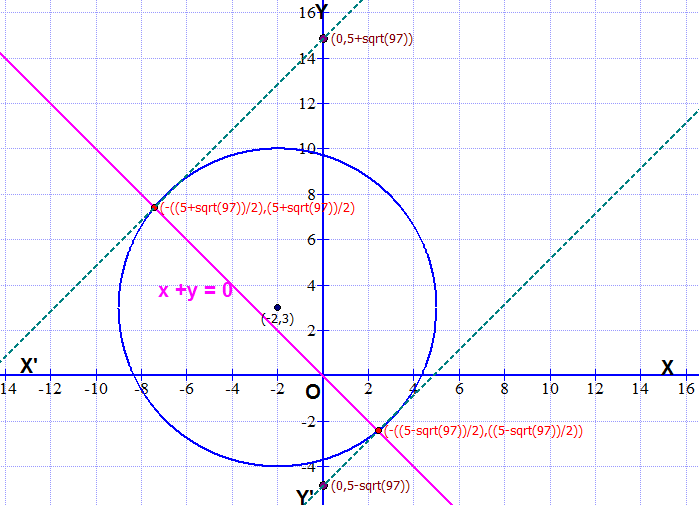
Equation of the straight line perpendicular to the sraight line
Let us solve for
Inserting
when
Now from given equation
we get
and
when
for this coordinate

