Question

Given a right triangle, let x be one of its acute angles. Suppose that the side opposite to angle x has length 15 and that the side adjacent to angle x has length 23. How do you find the approximate value of angle x in radians?

Answer 1

Start by drawing a diagram.

Explanation:

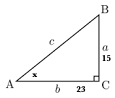
This is right angled trigonometry, so the technique to proceed is via SOHCAHTOA. First, out of `sin`, `cos` and `tan` we have to determine which trigonometric function to use.

Here are the definitions:

-`sintheta = "opposite"/"hypotenuse"`

-`costheta = "adjacent"/"hypotenuse"`

-`tantheta = "opposite"/"adjacent"`

These definitions are extremely important. They will serve you throughout your mathematical career in school. At the beginning, you can remember them with SOHCAHTOA, or Sin = opposite/hypotenuse, etc.

The next step in determining the value of `x` is seeing what sides we know. It is clearly stated in the problem we know the side opposite `x` and the side adjacent `x`. Therefore, we use tangent.

Now, we must set up a proportion.

`tanx = "opposite"/"adjacent"`

`tanx = 15/23`

`x = tan^(-1)(15/23)` Note: This can also be noted as `x = arctan(15/23)`

`x =0.58"radians"`

Hopefully this helps!