Help? Kinematics: Projectile motion
A cannon is positioned on an inclined plane with angle #alpha# . Find angle #beta# . We want a projectile fired by cannon to hit inclined plane with angle 90°
see picture
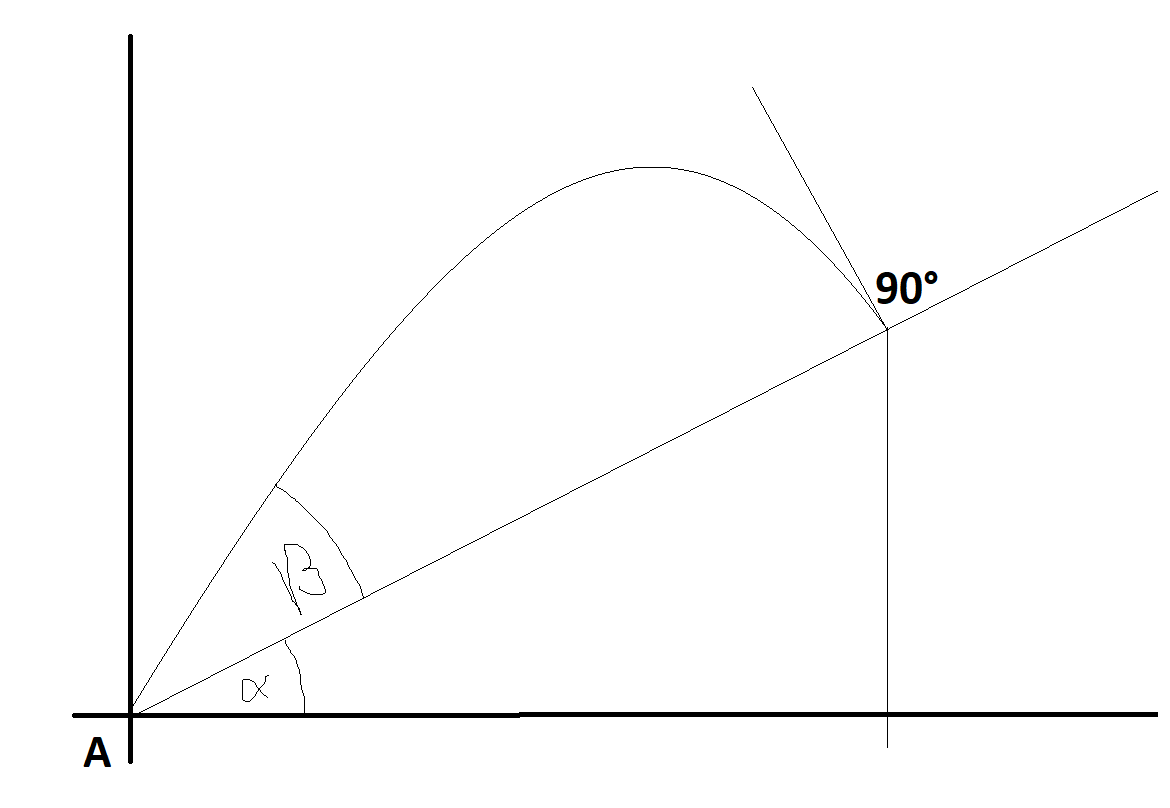
A cannon is positioned on an inclined plane with angle
see picture

1 Answer
Explanation:
It is easiest to solve this problem if we set up an coordinate system with the origin at the point of projection, the
In this coordinate system, both the
Quick check: this reduces to the familiar
Thus we have
and
According to the problem, when the projectile hits the plane on its way down (
while
Equating these two values for

