How do you determine the length of x in a right triangle when some of the information given is not part of the right triangle in question, but instead part of an outside acute angle? (Image included)
Here is the problem I am trying to figure out. I know I can use the 38° and 100 somehow, but since it isn't a right angle I am not sure how.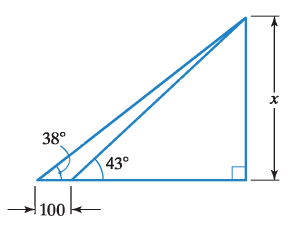
Here is the problem I am trying to figure out. I know I can use the 38° and 100 somehow, but since it isn't a right angle I am not sure how.
1 Answer
Explanation:
From what I can see, there is now way that we can solve this using only right triangles. Perhaps other contributors will find a way to use only right triangles, but I have a different approach to suggest.
The angle to the left of the
Let the last unknown angle in the oblique triangle be
We now know the angle opposite the only side we know as well as the angle opposite the hypotenuse (in the oblique triangle). We will therefore use the Law of Sines to determine the length of the hypotenuse of the right triangle.
Let the hypotenuse of the right triangle be
We'll keep it in exact value for now because at the end we want to have the most precise answer as possible.
We can now set up our ratio. We want to determine the length of
Hopefully this helps!

