You start by assuming the equation defines #y# as a function of #x# (the method we're about to use is called "implicit differentiation" because #y# has not been solved for explicitly in terms of #x#). Then you differentiate both sides with respect to #x#, using the Product Rule and Chain Rule to get the following (using Lebniz notation):
#y^2+2xy\frac{dy}{dx}+y+x\frac{dy}{dx}=0.#
This is an algebra equation that you can now solve for the derivative #dy/dx# to get:
#\frac{dy}{dx}=\frac{-y^{2}-y}{2xy+x}.#
If this is confusing, it might be less confusing if you write #y=f(x)# and then write the original equation as #x(f(x))^2+xf(x)=12#. Now when you differentiate both sides with respect to #x#, you'd write the initial result as
#(f(x))^2+2xf(x)f'(x)+f(x)+xf'(x)=0#
and then solve for #f'(x)# to get
#f'(x)=\frac{-(f(x))^2-f(x)}{2xf(x)+x}.#
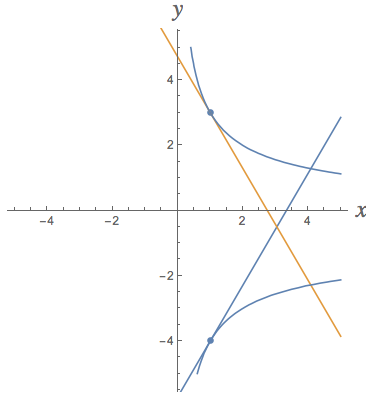
To use this derivative to find the slope of the curve at a specific value of #x#, you'd also have to find a corresponding value of #y# and plug both numbers into the equation for the derivative. For example, if #x=1#, then the original equation becomes #y^2+y=12# or #y^2+y-12=0# or #(y+4)(y-3)=0#, giving two corresponding values of #y#: #y=-4# and #y=3#.
The slope of the curve at #(x,y)=(1,-4)# would be #\frac{dy}{dx}=\frac{-(-4)^2-(-4)}{2\cdot 1\cdot (-4)+1}=\frac{-12}{-7}=\frac{12}{7}# and the slope of the curve at #(x,y)=(1,3)# would be #\frac{dy}{dx}=\frac{-(3)^2-(3)}{2\cdot 1\cdot (3)+1}=\frac{-12}{7}=-\frac{12}{7}#.
Here's a picture of this situation: