Question

How do you factor `x^4-61x^2+900`?

Answer 1

`(x+5)(x-5)(x+6)(x-6)`

Explanation:

`x^4-61x^2+900 = x^4-(25+36)x^2+900`

`rArrx^4-25x^2-36x^2+900`

`rArr x^2(x^2-25)-36(x^2-25)`

`rArr(x^2-25)(x^2-36)`

`rArr (x^2-5^2)(x^2-6^2)` [here applying `a^2-b^2=(a-b)(a+b)`]

`rArr (x+5)(x-5)(x+6)(x-6)`

Answer 2

`=(x+5)(x-5)(x+6)(x-6)`

Explanation:

Finding the correct factors for a quadratic trinomial which has a big value for (c) is not as daunting as it might appear at first:

There are a number of clues to look out for.

In `ax^2 + bx +c` , if `a=1`, then the difference in size between `b and c` is important. If `b` is quite small it means that the factors you are looking for are close to `sqrtc`

If `b` is quite large and almost the same size as `c`, then you are working with one very big and one very small factor.

Also remember that an odd number can only come from
ODD + EVEN, so this immediately eliminates the situation of two even factors.

`61` is quite small compared to `900`

`sqrt900 = 30 and 30 +30 = 60`

Therefore the required factors are not very far from `30` and must be odd and even

Consider factors less than `30` for divisibility into `900`.

`29? 28? 27? 26? 25?`

Ah, `25` seems possible.

`900 div 25 = 36" and "25 +36 = 61" "`
so these are the factors we want.

`x^4 -61x^2 +900`

`=(x^2 -25)(x^2-36)`

`=(x+5)(x-5)(x+6)(x-6)`

Answer 3

A difference approach

`=>x^4-61x^2+900 " "=" "(x-6)(x+6)(x-5)(x+5)=0`

Explanation:

Given:`" "x^4-61x^2+900`

Set `" "x^4-61x^2+900=0`

Set `beta =x^2` then by substitution we have:

`beta^2-61beta+900=0`

compare to `y=ax^2+bx+c`

`beta=(-b+-sqrt(b^2-4ac))/(2a)`

where `x^2=beta"; "a=1"; "b=-61"; "c=+900`

`x^2=(61+-sqrt((-61)^2-4(1)(900)))/(2(1))`

`x^2=61/2+-11/2`

`x^2=36 and 25`

`x=+-6 and +-5`

`=>x^4-61x^2+900 " "=" "(x-6)(x+6)(x-5)(x+5)`

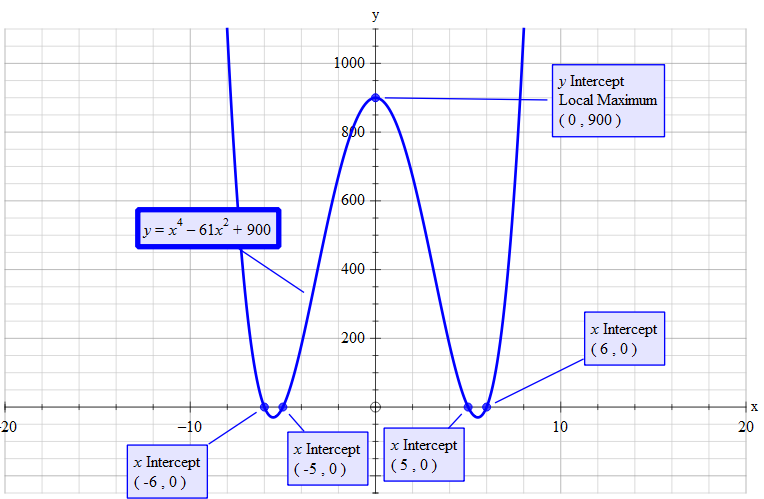
Note about the minimums.

You must not assume that they are centrally located between the points where the plot crosses the x-axis. In fact if you differentiate and set it to 0 you will find that they are slightly off centre.

`dy/dx=4x^3-122x=0`

`=>x=0 and [+-sqrt(30.5)~~+-5.523" to 3 decimal places"]`

Answer 4

`(x - 5)(x + 5)(x - 6)(x + 6)`

Explanation:

Call `X = x^2`, and factor this trinomial:

`f(X) = X^2 - 61X + 900`.

Find 2 numbers knowing the sum `(b = - 61)`,
and the product (`c = 900)`.

To do so, compose factor pairs of `(900)`

`rarr: ...(-18, -50);(-20, -45);(-25, -36).`

This last sum is `(-25 - 36 = - 61 = - b).`

Therefore. the 2 numbers are: `- 25 and - 36.`

`f(X) = (X - 25)(X - 36).`

Replace `X " by " x^2`

`f(x) = (x^2 - 25)(x^2 - 36) = (x - 5)(x + 5)(x - 6)(x + 6)`