Question

How do you find a standard form equation for the line with (-1,-2) ; perpendicular to the line 2x+5y+8=0?

Answer 1

`2y-5x-1=0`
or
`5x-2y+1=0`

Explanation:

Always keep in mind that when two lines are perpendicular, the product of their slopes is -1.

Proof :-

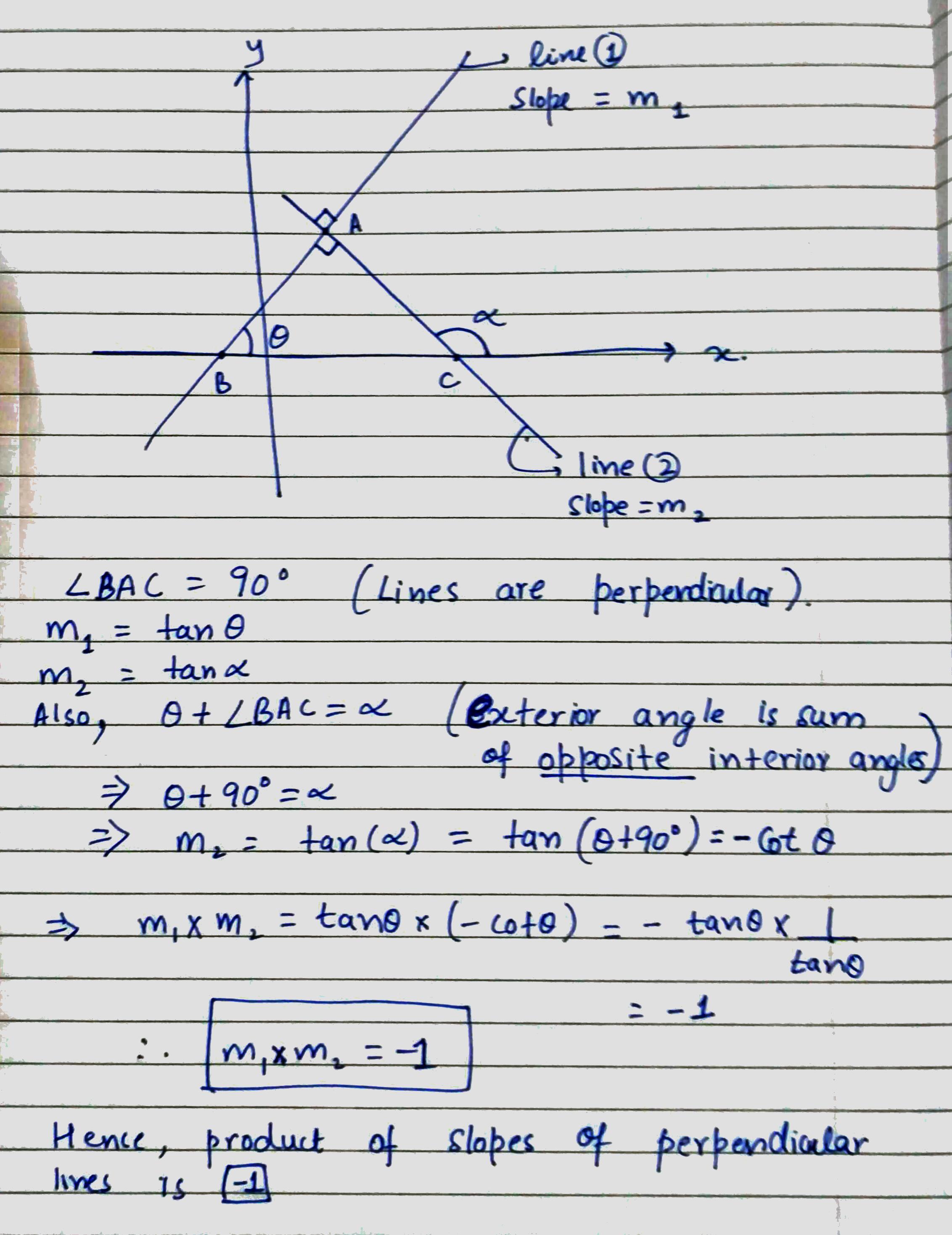
slope intercept equation of a straight line is given as:-
`y = mx+c`
`->` `m=` slope
`->` `c=` y intercept (y coordinate of the point where the line intersects the y axis) which is a constant for a given line.

given line `2x+5y+8=0` can be written as
`5y=-8-2x` `=>` `y=-2/5x - 8/5`
comparing with slope intercept form, slope `m_1=-2/5`
let slope of second line be `m_2`.
`therefore` `m_1*m_2 = -1` (given lines are perpendicular)
`implies m_2 = 5/2`

`therefore` equation of line we have to find is given by:-

`y=5/2x+c_2` where

since it passes through `(-1,-2)` substituting this point in the equation,
`-2=5/2(-1)+c_2`
`implies c_2= 5/2-2 = 1/2`

hence equation becomes,
`y=5/2x+1/2`
`i.e. 2y-5x-1=0`

Hence required equation is `2y-5x-1=0` or `5x-2y+1=0`